Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Notes

See Related Pages\(\)
\(\bullet\text{ Statistics Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Least Squares Regression Line}\)
\(\,\,\,\,\,\,\,\,\hat{y}=\overline{y}+b_1 \left( x-\overline{x} \right) \)
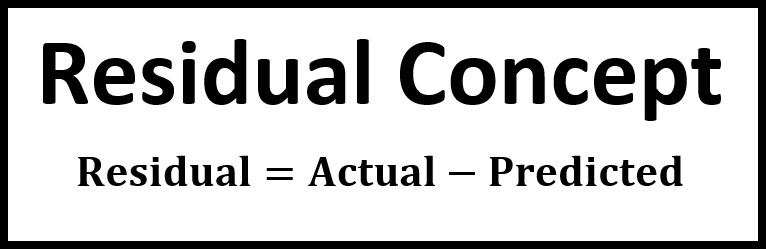
\(\bullet\text{ Residuals}\)
\(\,\,\,\,\,\,\,\,\text{Residual}=y-\hat{y}\)
\(\bullet\text{ Correlation Coefficient}\)
\(\,\,\,\,\,\,\,\,r=\displaystyle\frac{1}{n-1}\sum \left(\frac{x_i-\overline{x}}{s_x}\right)\left(\frac{y_i-\overline{y}}{s_y}\right)\)
\(\bullet\text{ Coefficient of Determination}\)
\(\,\,\,\,\,\,\,\,r^2=\displaystyle\frac{VAR(\hat{y})}{VAR(y)}\)
In Summary
In statistics, a residual is the difference between the observed value of a data point and the predicted value of that data point. In other words, it is the vertical distance between the data point and the line of best fit. For example, if a model predicts that a person’s height will be 70 inches, but the person’s actual height is 72 inches, the residual would be 2 inches.
Residuals, also known as errors or residual errors, are a key concept in statistical analysis. They are used to evaluate the accuracy of a model. If the residuals are randomly distributed around zero, it indicates that the model is a good fit for the data. If the residuals are not randomly distributed, it indicates that the model is not a good fit for the data and needs to be revised.
By analyzing the residuals, we can determine whether a model is overfitting or underfitting the data, and make adjustments to improve the model’s performance. Additionally, residuals can help identify outliers or errors in the data, and allow us to make more informed decisions based on the results of our statistical analysis.
Residuals are typically introduced in an introductory statistics course, which is often a required course for students majoring in fields such as math, economics, and psychology. In this course, students learn the basics of statistical analysis, including how to calculate and interpret residuals.
Residuals were first introduced by the statistician Carl Friedrich Gauss in the early 19th century. Gauss was a mathematician and astronomer who made significant contributions to a wide range of fields, including mathematics, physics, and astronomy. Gauss used residuals as a way to evaluate the accuracy of statistical models, and his work laid the foundation for modern statistical analysis.
real world examples of residuals in statistics
In medical research, residuals can be used to determine the effectiveness of a new treatment or medication. For example, if a group of patients is given a new drug to treat a certain condition, the residuals can be used to compare the improvement in their symptoms to the improvement in a control group that did not receive the treatment.
In economics, residuals can be used to analyze the relationship between different variables, such as GDP and unemployment rate. By calculating the residuals, economists can identify any factors that may not be accounted for in the model, such as changes in government policies or technological advancements.
In the field of education, residuals can be used to analyze the effectiveness of different teaching methods or instructional materials. For example, a school district may compare the test scores of students who received a new curriculum with those of students who did not, in order to determine the impact of the curriculum on student performance.
In meteorology, residuals can be used to evaluate the accuracy of weather forecasts. By comparing the predicted weather conditions with the actual conditions that occurred, meteorologists can determine the extent to which the forecast was accurate and identify any factors that may have contributed to any discrepancies.
In the field of finance, residuals can be used to evaluate the performance of investment portfolios. By comparing the returns on a portfolio to the returns of a benchmark index, such as the S&P 500, investors can determine the extent to which the portfolio outperformed or underperformed the benchmark.
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!