Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Practice Problems
\(\textbf{1)}\) Are angles 1 and 2 adjacent?
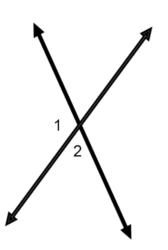
\(\textbf{2)}\) Are angles 1 and 2 adjacent?
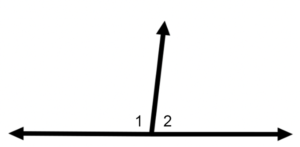
\(\textbf{3)}\) Are angles 1 and 2 adjacent?
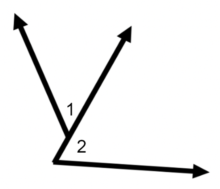
\(\textbf{4)}\) Are angles 1 and 2 adjacent?
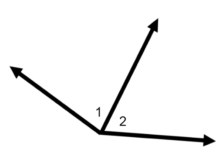
\(\textbf{5)}\) Are angles 1 and 2 adjacent?
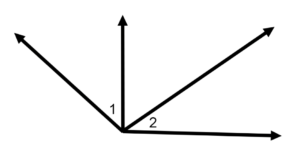
\(\textbf{6)}\) Are angles 1 and 2 adjacent?
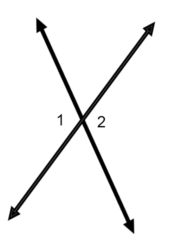
True or False?
\(\textbf{7)}\) Adjacent angles can be supplementary.
\(\textbf{8)}\) All Vertical angles are adjacent angles.
See Related Pages\(\)
\(\bullet\text{ Geometry Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Angle Addition Postulate}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Complimentary and Supplementary Angles}\)
\(\,\,\,\,\,\,\,\,\)
In Summary
Adjacent angles are two angles that share a common side and a common vertex, or point of intersection. They are called “adjacent” because they are next to each other. Adjacent angles are typically introduced in geometry classes in middle or high school. They are a basic concept in geometry and are often covered in early lessons on angles and angle relationships. Adjacent angles are also a foundational concept for more advanced topics in geometry, such as parallel lines and transversals, and they may be revisited and built upon in later lessons.
In addition to being introduced in geometry classes, adjacent angles may also be covered in other math classes that involve geometry, such as algebra and trigonometry. For example, students may use their knowledge of adjacent angles to solve equations or to understand the properties of triangles and other geometric figures.
The most common mistakes with adjacent angles is misinterpreting the definition of adjacent angles. Some students might confuse adjacent angles with angles that are simply next to each other, rather than angles that share a common side and a common vertex.
A linear pair is a formal name for a pair of adjacent angles that are supplementary.
Adjacent angles have been studied and understood by mathematicians for thousands of years. The concept of adjacent angles can be traced back to ancient Greek geometry, where it was studied by mathematicians such as Euclid and Pythagoras. Euclid, in particular, is known for his contributions to the study of geometry, including the concept of adjacent angles. In his influential work “Elements,” Euclid provided a rigorous treatment of geometric concepts, including the definition and properties of adjacent angles. It’s worth noting that the concept of adjacent angles is a fundamental concept in geometry, and it is likely that it was understood by mathematicians in other ancient civilizations as well. However, the specific history and origins of the concept may vary depending on the culture and the specific mathematical traditions that were studied.
Topics related to adjacent angles
Complementary and supplementary angles: As mentioned earlier, adjacent angles can be either complementary (adding up to 90 degrees) or supplementary (adding up to 180 degrees). Understanding the difference between complementary and supplementary angles, and how to recognize and work with them, is an important skill when working with adjacent angles.
Linear pairs: Linear pairs are pairs of adjacent angles that add up to 180 degrees. In other words, linear pairs are supplementary angles that are also adjacent. Understanding linear pairs can help you recognize and work with adjacent angles in a variety of contexts.
Vertical angles: Vertical angles are pairs of angles that are opposite each other and formed by two intersecting lines. These angles are not adjacent, but they can be related to adjacent angles in certain situations. For example, if two lines intersect and form a linear pair of adjacent angles, the vertical angles will also be supplementary.
Parallel lines and transversals: Adjacent angles can be used to understand the relationship between parallel lines and transversals, which are lines that intersect at an angle. When two lines are parallel, the pairs of adjacent angles formed by the intersection with a transversal are called corresponding angles. These angles are always congruent (meaning they have the same measure).
Triangles and other geometric figures: Adjacent angles can also be used to understand the properties of triangles and other geometric figures. For example, the sum of the interior angles of a triangle is always 180 degrees, and adjacent angles in a triangle can be used to help prove this fact.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!