Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Lesson
Notes
Practice Problems
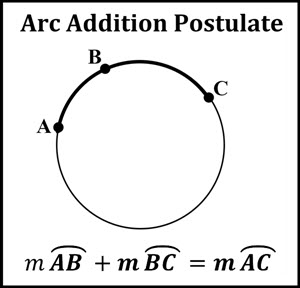
\(\textbf{1)}\) If \(m\stackrel \frown{AB}=40^{\circ}\) and \(m\stackrel \frown{BC}=60^{\circ}\), what is \(m\stackrel \frown{AC}\)?
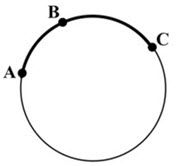

\(\textbf{2)}\) If \(m\stackrel \frown{AB}=38^{\circ}\) and \(m\stackrel \frown{AC}=130^{\circ}\), what is \(m\stackrel \frown{BC}\)?

\(\textbf{3)}\) If \(m\stackrel \frown{AB}=(4x+8)^{\circ}\) , \(m\stackrel \frown{BC}=(6x+12)^{\circ}\), and \(m\stackrel \frown{AC}=120^{\circ}\) what is \(m\stackrel \frown{AB}\)?

See Related Pages\(\)
\(\bullet\text{ Geometry Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Segment Addition Postulate}\)
\(\,\,\,\,\,\,\,\,AB+BC=AC…\)
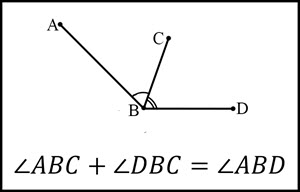
\(\bullet\text{ Angle Addition Postulate}\)
\(\,\,\,\,\,\,\,\,\)
In Summary
The Arc Addition Postulate is a fundamental principle in the study of geometry, specifically in the study of circles and arcs. The arc addition postulate states that if two points, A and B, are connected by an arc and a third point, C, lies on this arc, then the length of the arc from A to B is equal to the sum of the lengths of the arcs from A to C and from C to B. In other words, if we have an arc that is made up of two smaller arcs that are connected end-to-end, then the length of the larger arc is equal to the sum of the lengths of the smaller arcs. This principle holds true no matter where point C is located on the arc between points A and B.
The Arc Addition Postulate is typically introduced in a high school or college level geometry course. It is a fundamental principle that is used throughout the study of geometry, and is often discussed in the context of circles and arcs. In a high school geometry course, the Arc Addition Postulate may be introduced in a unit on circles and arcs, along with other principles such as the Inscribed Angle Theorem. In a college level course, the Arc Addition Postulate may be discussed as part of a more comprehensive study of geometric principles and techniques.
The Arc Addition Postulate is a fundamental principle in geometry that has been known and studied for centuries. It is not known who first discovered or formulated the postulate, as it is a basic principle that has likely been understood for as long as people have been studying geometry.
The principles of geometry have been studied by mathematicians and scholars in many different cultures and time periods throughout history. The Arc Addition Postulate is a simple and intuitive principle that is likely to have been discovered and understood independently by many different people over the course of history.
The Arc Addition Postulate is now a standard part of the geometry curriculum in schools and is widely understood and used by mathematicians and professionals in a variety of fields.
Topics related to Arc Addition Postulate
Circle Postulate: The Circle Postulate is a fundamental principle in geometry that states that every point on the circumference of a circle is an equal distance from the center of the circle.
Inscribed Angle Theorem: The Inscribed Angle Theorem states that if an angle is inscribed in a circle (meaning that its vertex is on the circumference of the circle and its sides pass through the center of the circle), then the measure of the angle is half the measure of the arc it intercepts on the circumference of the circle. This theorem is often used in conjunction with the Arc Addition Postulate to solve problems involving inscribed angles and arcs.
Chord Length: A chord is a line segment that connects two points on the circumference of a circle. The length of a chord is an important concept in geometry, and it can be related to the measure of the arc it intercepts.
Central Angle: A central angle is an angle formed by two radii of a circle that connect the center of the circle to two points on the circumference. The measure of a central angle is equal to the measure of the arc it intercepts on the circumference of the circle.
These are just a few examples of the topics that are related to the Arc Addition Postulate in the study of geometry. Understanding these principles and how they relate to each other can help you more effectively solve problems involving arcs and angles in circles.
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!