\(\textbf{1)}\) Find \( \displaystyle \lim_{x\to 2^{-}} f(x) \)
where \(f(x) = \begin{cases}
5x+3 & \text{if } x \lt 2 \\
4x & \text{if } x \geq 2
\end{cases}\)

Show Answer

The limit is \( 13 \)

\(\textbf{2)}\) Find \( \displaystyle \lim_{x\to 2^{+}} f(x) \)
where \(f(x) = \begin{cases}
5x+3 & \text{if } x \lt 2 \\
4x & \text{if } x \geq 2
\end{cases}\)

Show Answer

The limit is \( 8 \)

\(\textbf{3)}\) \( \displaystyle \lim_{x\to 2^{+}} \frac{|x-2|}{x-2} \)

Show Answer

The answer is \( 1 \)

Show Work

Let’s start by evaluating the expression for values of \(x\) just to the right of 2.

For \(x = 2.1\), \(\frac{|2.1 – 2|}{2.1 – 2} = \frac{0.1}{0.1} = 1\)

For \(x = 2.01\), \(\frac{|2.01 – 2|}{2.01 – 2} = \frac{0.01}{0.01} = 1\)

For \(x = 2.001\), \(\frac{|2.001 – 2|}{2.001 – 2} = \frac{0.001}{0.001} = 1\)

As \(x\) approaches 2 from the right, the expression \(\frac{|x-2|}{x-2}\) approaches 1.

The answer is \( 1 \)

\(\textbf{4)}\) \( \displaystyle \lim_{x\to 2^{-}} \frac{|x-2|}{x-2} \)

Show Answer

The answer is \( -1 \)

Show Work

Let’s start by evaluating the expression for values of \(x\) just to the left of 2.

For \(x = 1.9\), \(\frac{|1.9 – 2|}{1.9 – 2} = \frac{0.1}{0.1} = -1\)

For \(x = 1.99\), \(\frac{|1.99 – 2|}{1.99 – 2} = \frac{0.01}{0.01} = -1\)

For \(x = 1.999\), \(\frac{|1.999 – 2|}{1.999 – 2} = \frac{0.001}{0.001} = -1\)

As \(x\) approaches 2 from the left, the expression \(\frac{|x-2|}{x-2}\) approaches -1.

The answer is \( -1 \)

\(\textbf{5)}\) \( \displaystyle \lim_{x\to 2} \frac{|x-2|}{x-2} \)

Show Answer

The Limit Does Not Exist

Show Work

Let’s start by evaluating the expression for values of \(x\) just to the right and left of 2.

For \(x = 1.9\), \(\frac{|1.9 – 2|}{1.9 – 2} = \frac{0.1}{0.1} = -1\)

For \(x = 2.1\), \(\frac{|2.1 – 2|}{2.1 – 2} = \frac{0.1}{0.1} = 1\)

As \(x\) approaches 2 from both sides, the expression \(\frac{|x-2|}{x-2}\) approaches \(\pm 1\).
Therefore, the limit does not exist.

\(\textbf{6)}\) \( \displaystyle \lim_{x\to 1^{+}} \frac{3|x-1|}{x-1} \)

Show Answer

The answer is \( 3 \)

Show Work

Let’s start by evaluating the expression for values of \(x\) just to the right of 1.

For \(x = 1.1\), \(\frac{3|1.1 – 1|}{1.1 – 1} = \frac{3|0.1|}{0.1} = 3\)

For \(x = 1.01\), \(\frac{3|1.01 – 1|}{1.01 – 1} = \frac{3|0.01|}{0.01} = 3\)

For \(x = 1.001\), \(\frac{3|1.001 – 1|}{1.001 – 1} = \frac{3|0.001|}{0.001} = 3\)

As \(x\) approaches 1 from the right, the expression \(\frac{3|x-1|}{x-1}\) approaches 3.

The answer is \( 3 \)

\(\textbf{7)}\) \( \displaystyle \lim_{x\to 1^{-}} \frac{3|x-1|}{x-1} \)

Show Answer

The answer is \( -3 \)

Show Work

Let’s start by evaluating the expression for values of \(x\) just to the left of 1.

For \(x = 0.9\), \(\frac{3|0.9 – 1|}{0.9 – 1} = \frac{3|-0.1|}{-0.1} = -3\)

For \(x = 0.99\), \(\frac{3|0.99 – 1|}{0.99 – 1} = \frac{3|-0.01|}{-0.01} = -3\)

For \(x = 0.999\), \(\frac{3|0.999 – 1|}{0.999 – 1} = \frac{3|-0.001|}{-0.001} = -3\)

As \(x\) approaches 1 from the left, the expression \(\frac{3|x-1|}{x-1}\) approaches -3.

The answer is \( -3 \)

\(\textbf{8)}\) \( \displaystyle \lim_{x\to 1} \frac{3|x-1|}{x-1} \)

Show Answer

The Limit Does Not Exist

Show Work

Let’s start by evaluating the expression for values of \(x\) just to the right and left of 1.

For \(x = 0.9\), \(\frac{3|0.9 – 1|}{0.9 – 1} = \frac{3|-0.1|}{-0.1} = -3\)

For \(x = 1.1\), \(\frac{3|1.1 – 1|}{1.1 – 1} = \frac{3|0.1|}{0.1} = 3\)

As \(x\) approaches 1 from both sides, the expression \(\frac{3|x-1|}{x-1}\) approaches \(\pm 3\).
Therefore, the limit does not exist.

\(\textbf{9)}\) Find \( \displaystyle \lim_{x\to 4^{-}} f(x) \)
where \(f(x) = \begin{cases}
-x+5 & \text{if } x\leq 4 \\
x-3 & \text{if } 4\lt x \lt 6 \\
x & \text{if }x\geq 6
\end{cases}\)

Show Answer

The limit is \( 1 \)

\(\textbf{10)}\) Find \( \displaystyle \lim_{x\to 4^{+}} f(x) \)
where \(f(x) = \begin{cases}
-x+5 & \text{if } x\leq 4 \\
x-3 & \text{if } 4\lt x \lt 6 \\
x & \text{if }x\geq 6
\end{cases}\)

Show Answer

The limit is \( 1 \)

\(\textbf{11)}\) Find \( \displaystyle \lim_{x\to 6^{-}} f(x) \)
where \(f(x) = \begin{cases}
-x+5 & \text{if } x\leq 4 \\
x-3 & \text{if } 4\lt x \lt 6 \\
x & \text{if }x\geq 6
\end{cases}\)

Show Answer

The limit is \( 3 \)

\(\textbf{12)}\) Find \( \displaystyle \lim_{x\to 6^{+}} f(x) \)
where \(f(x) = \begin{cases}
-x+5 & \text{if } x\leq 4 \\
x-3 & \text{if } 4\lt x \lt 6 \\
x & \text{if }x\geq 6
\end{cases}\)

Show Answer

The limit is \( 6 \)

\(\textbf{13)}\) Find \( \displaystyle \lim_{x\to 7^{-}} f(x) \)
where \(f(x) = \begin{cases}
-x+5 & \text{if } x\leq 4 \\
x-3 & \text{if } 4\lt x \lt 6 \\
x & \text{if }x\geq 6
\end{cases}\)

Show Answer

The limit is \( 7 \)

\(\textbf{14)}\) Find \( \displaystyle \lim_{x\to 7^{+}} f(x) \)
where \(f(x) = \begin{cases}
-x+5 & \text{if } x\leq 4 \\
x-3 & \text{if } 4\lt x \lt 6 \\
x & \text{if }x\geq 6
\end{cases}\)

Show Answer

The limit is \( 7 \)

\(\textbf{15)}\) \( \displaystyle \lim_{x\to 1^{-}} \frac{x^2+3x-4}{\left(x-1\right)^4} \)

Show Answer

The answer is \(-\infty\)

See Related Pages\(\)

\(\bullet\text{ Calculus Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)

\(\bullet\text{ Limits on Graphs}\)
\(\,\,\,\,\,\,\,\,\)\(…\)

\(\bullet\text{ Continuity on Graphs}\)
\(\,\,\,\,\,\,\,\,\)\(…\)

\(\bullet\text{ Piecewise Functions- Limits and Continuity}\)
\(\,\,\,\,\,\,\,\,\)\(…\)

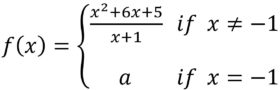\(\bullet\text{ Limits at Infinity}\)
\(\,\,\,\,\,\,\,\,\displaystyle\lim_{x\to \infty}\frac{5x^2+2x-10}{3x^2+4x-5}…\)

\(\bullet\text{ Trig Limits}\)
\(\,\,\,\,\,\,\,\,\displaystyle \lim_{\theta\to0} \frac{\sin \theta}{\theta}=1…\)

In Summary

One-sided limits are a type of mathematical concept defined as the limit of a function as it approaches a certain value from one direction, either from the left or the right. In order for a limit to exist at a point, both the left and right limits must be the same. One of the main reasons we learn about one-sided limits is because for a lot of functions, the left limit doesn’t always equal the right limit.

One-sided limits are typically introduced in calculus courses.

One-sided limits were first formally introduced by Augustin-Louis Cauchy in the early 19th century.

About Andymath.com

Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.

Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.

Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!

Thank you for visiting. How exciting!