Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
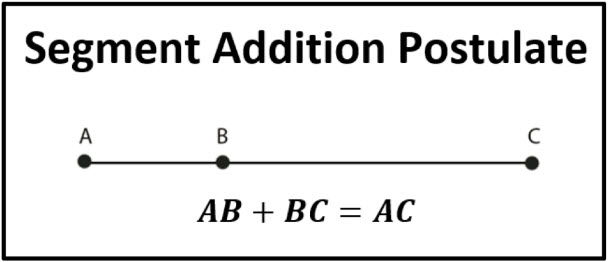
The segment addition postulate is a formal way of saying the two parts add up to make the whole.
It applies to adjacent segments lying on the same line.
Notes
Problems
\(\textbf{1)}\) If AB\(=10\) and BC\(=25\), what is AC?


\(\textbf{2)}\) If AC\(=40\) and BC\(=18\), what is AB?

\(\textbf{3)}\) If AC\(=52\) and AB\(=10\), what is BC?

\(\textbf{4)}\) If BC\(=28\) and AB\(=9\), what is AC?

\(\textbf{5)}\) What is the length of AC as an expression?
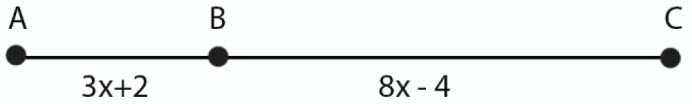

\(\textbf{6)}\) If AC\(=10x-4\), what is BC as an expression?


\(\textbf{7)}\) B is the midpoint of AC. (Not drawn to scale) If AB\(=52\) and AC\(=4x+4\), what is the value of x?

See Related Pages\(\)
\(\bullet\text{ Geometry Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
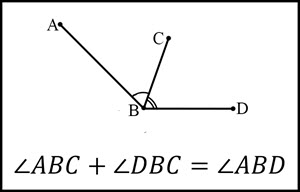
\(\bullet\text{ Angle Addition Postulate}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Complimentary and Supplementary Angles}\)
\(\,\,\,\,\,\,\,\,\)
In Summary
The segment addition postulate is a fundamental principle in geometry that states that given two line segments with a common endpoint and slope, the sum of the lengths of the two segments is equal to the length of the line segment formed by the two segments taken together. This principle is often used to find the lengths of unknown segments in a figure by adding known segments together. The segment addition postulate is a key concept in geometry and is used in various geometric proofs and constructions. Understanding this principle is important for further study in geometry and other areas of mathematics.
The segment addition postulate is a fundamental principle in geometry that has been known and used for thousands of years. It is likely that this principle was discovered and utilized by ancient mathematicians and geometers, who used it to solve problems and make measurements. The history of geometry can be traced back to ancient civilizations in Egypt, Mesopotamia, and Greece, where mathematicians and scholars developed the first geometric concepts and principles. Many of the fundamental principles of geometry, including the segment addition postulate, are attributed to the ancient Greek mathematician Euclid, who is considered the father of geometry. Euclid’s work, “The Elements,” is a collection of geometric definitions, theorems, and proofs that has had a profound influence on mathematics and geometry for over two thousand years. In modern times, the segment addition postulate is still an important principle in geometry and is taught to students in high school and college. It continues to be used in various fields, including engineering, architecture, and surveying, to solve problems and make measurements.
Real world examples of Segment Addition Postulate
Measuring the distance between two points: The segment addition postulate can be used to measure the distance between two points on a map or in a physical space. For example, consider a map of a city with two points A and C. To find the distance between A and C, we can use the segment addition postulate to add up the lengths of the segments that make up the path from A to C.
Designing a building or a bridge: Architects and engineers often use the segment addition postulate when designing buildings and bridges. For example, when designing a bridge, engineers may use the segment addition postulate to determine the length of the main span (the distance between the two endpoints of the bridge) by adding up the lengths of the smaller segments that make up the span.
Survey work: Land surveyors use the segment addition postulate to measure distances and angles in the field. For example, they may use a surveyor’s wheel or a laser rangefinder to measure the lengths of segments and use the segment addition postulate to find the total distance between two points.
Navigation: The segment addition postulate can also be used in navigation to find the distance between two points. For example, when using a GPS device, the device may use the segment addition postulate to add up the lengths of the segments between two points to calculate the total distance.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!