The Trapezoid Midsegment Theorem and the Converse can be used in many proofs involving trapezoids, and they can be used to solve for unknown values in trapezoids.

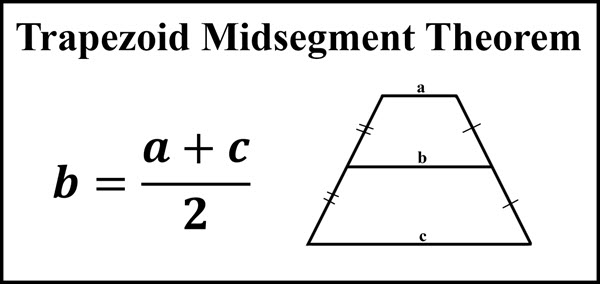
\(\bullet\) Trapezoid Midsegment Theorem
If a line segment connects the midpoints of the legs of the trapezoid, then it is parallel to the bases, and its length is equal to half the sum of the lengths of the bases \( \displaystyle \frac{b_1+b_2}{2}\)

\(\bullet\) Converse of Trapezoid Midsegment Theorem
If a line that is parallel to the bases of a trapezoid and intersects the midpoint of one of the legs, then it is a midsegment, it intersects the other leg’s midpoint, and its length is equal to half the sum of lengths of the bases. \( \displaystyle \frac{b_1+b_2}{2}\)

Notes

Practice Questions

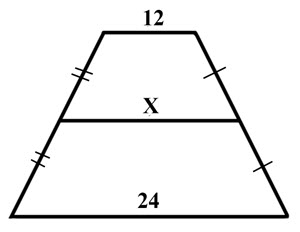
\(\textbf{1)}\) Solve for x.

Show Answer

\(\text{The answer is } x=18\)

Show Work

\(\,\,\,\,\,\,x=\displaystyle\frac{12+24}{2}\)

\(\,\,\,\,\,\,x=\displaystyle\frac{36}{2}\)

\(\,\,\,\,\,\,x=18\)

\(\textbf{2)}\) Solve for m.

Show Answer

\(\text{The answer is } m=14\)

Show Work

\(\,\,\,\,\,\,m=\displaystyle\frac{6+22}{2}\)

\(\,\,\,\,\,\,m=\displaystyle\frac{28}{2}\)

\(\,\,\,\,\,\,m=14\)

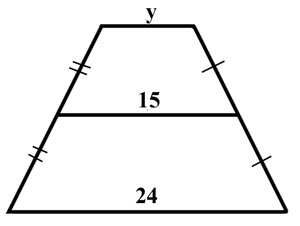
\(\textbf{3)}\) Solve for y.

Show Answer

\(\text{The answer is } y=6\)

Show Work

\(\,\,\,\,\,\,15=\displaystyle\frac{y+24}{2}\)

\(\,\,\,\,\,\,30=y+24\)

\(\,\,\,\,\,\,6=y\)

\(\,\,\,\,\,\,y=6\)

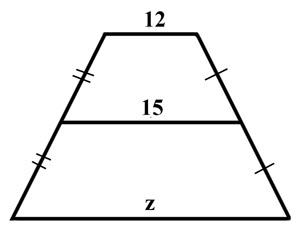
\(\textbf{4)}\) Solve for z.

Show Answer

\(\text{The answer is } z=18\)

Show Work

\(\,\,\,\,\,\,15=\displaystyle\frac{12+z}{2}\)

\(\,\,\,\,\,\,30=12+z\)

\(\,\,\,\,\,\,18=z\)

\(\,\,\,\,\,\,z=18\)

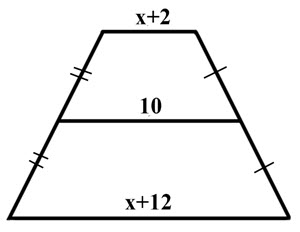
\(\textbf{5)}\) Solve for x.

Show Answer

\(\text{The answer is } x=3\)

Show Work

\(\,\,\,\,\,\,10=\displaystyle\frac{(x+2)+(x+12)}{2}\)

\(\,\,\,\,\,\,20=x+2+x+12\)

\(\,\,\,\,\,\,20=2x+14\)

\(\,\,\,\,\,\,6=2x\)

\(\,\,\,\,\,\,3=x\)

\(\,\,\,\,\,\,x=3\)

See Related Pages\(\)

\(\bullet\text{ Geometry Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)

\(\bullet\text{ Area of Trapezoids}\)
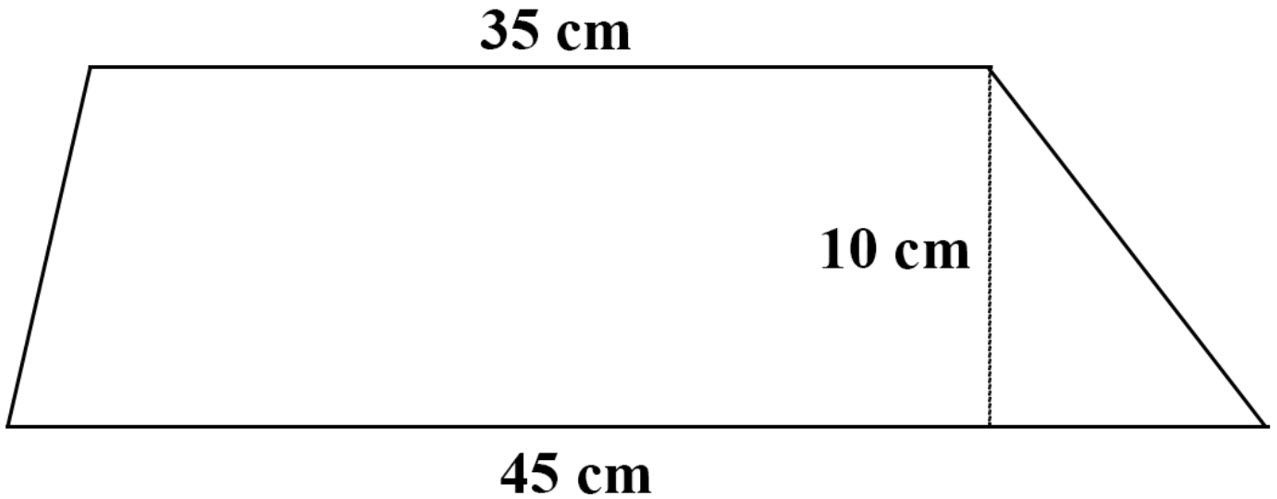\(\,\,\,\,\,\,\,\,\)\(A=\frac{1}{2}(b_1+b_2)\cdot h…\)

In Summary

A trapezoid is a four-sided polygon with one pair of parallel sides. The line that connects the midpoints of the non-parallel sides of a trapezoid is called the midsegment. The definition of a trapezoid midsegment is a line segment that lies in the middle of a trapezoid and connects the midpoints of the non-parallel sides of the trapezoid.

It is important to learn about trapezoid midsegments because they have several interesting properties and can be used in a variety of applications. Some properties are the midsegment of a trapezoid is parallel to the two bases (the parallel sides) the length is the average length of the two bases. Trapezoid midsegments are usually introduced in geometry classes, although they may also be covered in algebra and trigonometry courses.

It is not clear who first discovered the concept of trapezoid midsegments. Geometry has been studied for thousands of years, and the concept of a midsegment likely arose naturally as a way to describe and analyze the properties of trapezoids. Some related topics to trapezoid midsegments include parallel lines, midpoints, and trapezoids in general. Other related concepts might include medians, altitudes, and areas of trapezoids.

Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.

Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.

Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!

Thank you for visiting. How exciting!