Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Notes
Practice Problems
\(\textbf{1)}\) \( f(x)=\frac{3}{x}, \) find \( f'(x) \) using the definition of derivative
\(\textbf{2)}\) \( f(x)=3x^2+2x+1, \) find \( f'(x) \) using the definition of derivative
\(\textbf{3)}\) \( f(x)=x^2-3x+1, \) find \( f'(4) \) using the definition of derivative
\(\textbf{4)}\) \( f(x)=x^3-2x+1, \) find \( f'(x) \) using the definition of derivative
\(\textbf{5)}\) Find the instantaneous slope of \( f(x)=x^2+x \) at \( x=3 \) using the definition of derivative at a point.
See Related Pages\(\)
\(\bullet\text{ Calculus Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Definition of Derivative}\)
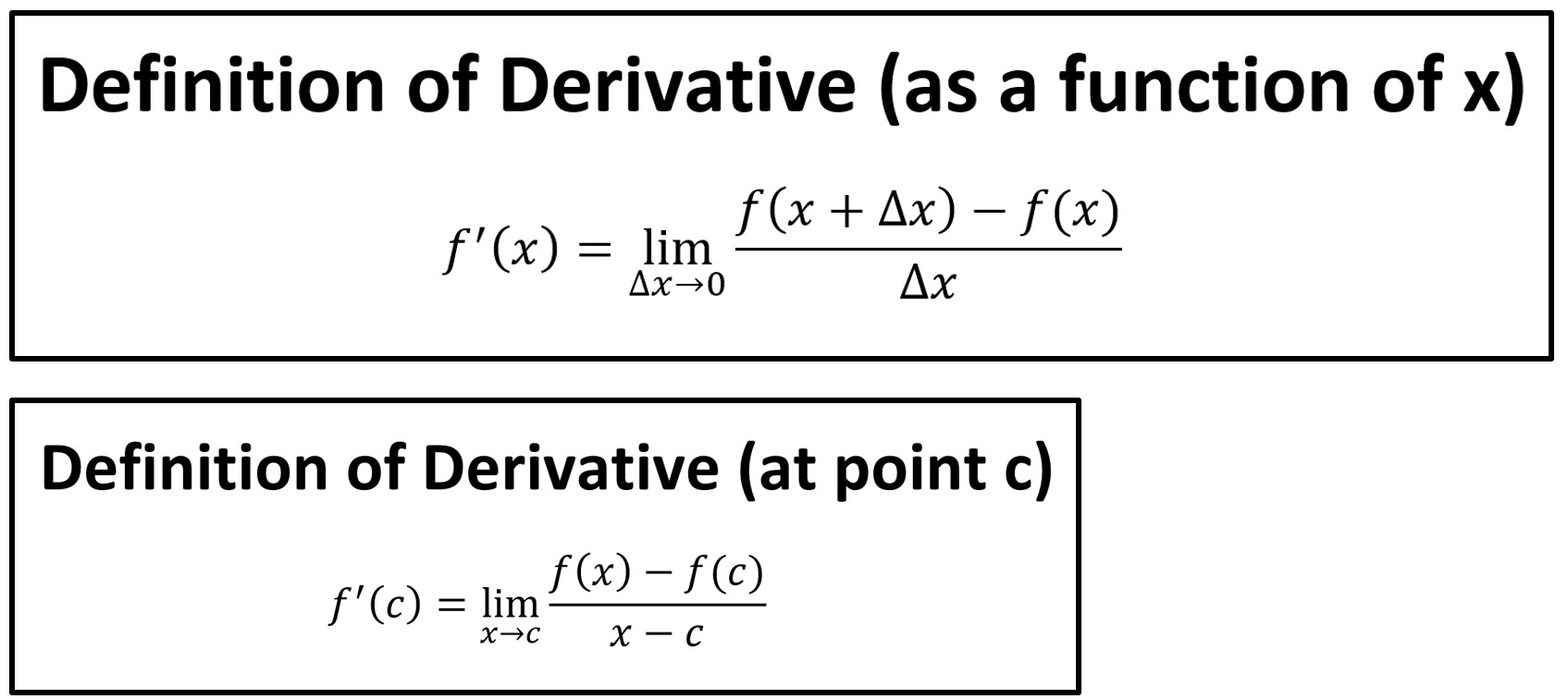
\(\,\,\,\,\,\,\,\, \displaystyle \lim_{\Delta x\to 0} \frac{f(x+ \Delta x)-f(x)}{\Delta x} \)
\(\bullet\text{ Equation of the Tangent Line}\)
\(\,\,\,\,\,\,\,\,f(x)=x^3+3x^2−x \text{ at the point } (2,18)\)
\(\bullet\text{ Derivatives- Constant Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(c)=0\)
\(\bullet\text{ Derivatives- Power Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(x^n)=nx^{n-1}\)
\(\bullet\text{ Derivatives- Constant Multiple Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(cf(x))=cf'(x)\)
\(\bullet\text{ Derivatives- Sum and Difference Rules}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(x) \pm g(x)]=f'(x) \pm g'(x)\)
\(\bullet\text{ Derivatives- Sin and Cos}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}sin(x)=cos(x)\)
\(\bullet\text{ Derivatives- Product Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(x) \cdot g(x)]=f(x) \cdot g'(x)+f'(x) \cdot g(x)\)
\(\bullet\text{ Derivatives- Quotient Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}\left[\displaystyle\frac{f(x)}{g(x)}\right]=\displaystyle\frac{g(x) \cdot f'(x)-f(x) \cdot g'(x)}{[g(x)]^2}\)
\(\bullet\text{ Derivatives- Chain Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(g(x))]= f'(g(x)) \cdot g'(x)\)
\(\bullet\text{ Derivatives- ln(x)}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[ln(x)]= \displaystyle \frac{1}{x}\)
\(\bullet\text{ Implicit Differentiation}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Horizontal Tangent Line}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Mean Value Theorem}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Related Rates}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Increasing and Decreasing Intervals}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Intervals of concave up and down}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Inflection Points}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Graph of f(x), f'(x) and f”(x)}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Newton’s Method}\)
\(\,\,\,\,\,\,\,\,x_{n+1}=x_n – \displaystyle \frac{f(x_n)}{f'(x_n)}\)
In Summary
A derivative is a mathematical concept that measures the rate of change of a function with respect to one of its variables. In other words, it describes how a function is changing as its input values change. Derivatives are commonly used in calculus, which is a branch of mathematics that deals with the study of rates of change and the accumulation of quantities. The definition of derivative can be formalized using the concept of limits.
Learning about derivatives is important for a variety of reasons. In particular, derivatives are used in many fields, including physics, engineering, economics, and finance, to model and analyze real-world phenomena. For example, derivatives can be used to calculate the rate at which a quantity is changingat a particular instant, such as the speed of a car or the rate at which a population is growing.
Derivatives are typically introduced in Calculus classes. The concept of derivatives was first formalized by Sir Isaac Newton and Gottfried Wilhelm Leibniz in the 17th century. Newton and Leibniz developed the mathematical principles of calculus independently of each other, and both are credited with the discovery of derivatives.
Some related topics to the derivatives include integrals, which are the opposite of derivatives and deal with the accumulation of quantities; optimization, which involves finding the maximum or minimum value of a function; and differential equations, which are equations that involve derivatives and are used to model many real-world phenomena.
Topics that use derivatives
Maxima and minima: The derivative of a function can be used to find the points at which a function has a maximum or minimum value. These points are called critical points, and they can be found by setting the derivative equal to zero and solving for the variables.
Optimization: The derivative of a function can be used to find the values of the variables that maximize or minimize the function. This is important in many fields, such as economics and engineering, where it is necessary to find the optimal solution to a problem.
Graph sketching: The derivative of a function can be used to determine the slope of the tangent line to the graph of the function at a given point. This can be used to sketch the graph of the function, by finding the points of inflection and concavity, and determining the shape of the graph near these points.
Taylor series: The derivative of a function can be used to find the terms of the Taylor series of the function, which is an infinite series that approximates the function. This is important in numerical analysis, where it is often necessary to approximate functions that cannot be solved exactly.
Differential equations: The derivative of a function is often used to define and solve differential equations, which are equations that involve an unknown function and its derivatives. Differential equations are used to model a wide range of phenomena in physics, engineering, and other fields.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!