Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Notes
Definition
Diagonal- a line segment connecting two nonadjacent vertices
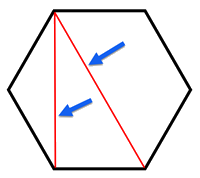
Number of Diagonals in a Polygon (n-gon)
\( \text{Diagonals} = \frac{n(n-3)}{2}\)
| \({\text{Number of diagonals in polygons}}\) | ||
| Triangle (3 sides) | No diagonals | 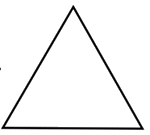 |
| Quadrilateral (4 sides) | 2 diagonals |  |
| Pentagon (5 sides) | 5 diagonals | 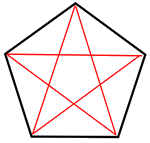 |
| Hexagon (6 sides) | 9 diagonals | 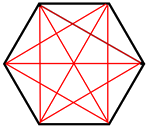 |
| Octagon (8 sides) | 20 diagonals | 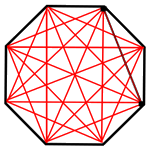 |
| N-gon (n sides) | \(\frac{n(n-3)}{2}\) diagonals | |
| \({\text{Properties of Diagonals of Quadrilaterals}}\) | |
| Parallelograms | Diagonals bisect each other |
| Rectangles | Diagonals bisect each other Diagonals are congruent |
| Rhombuses | Diagonals bisect each other Diagonals are perpendicular |
| Squares | Diagonals bisect each other Diagonals are perpendicular Diagonals are congruent |
| Kites | Diagonals are perpendicular to each other |
| Isosceles Trapezoids | Diagonals are Congruent |
See Related Pages\(\)
\(\bullet\text{ Geometry Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Intro to Polygons}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Area of a Regular Polygon (Apothem)}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Population Density}\)
\(\,\,\,\,\,\,\,\,\)
In Summary
Diagonals of polygons are line segments that connect two non-adjacent vertices of a polygon. They are different from the sides of the polygon, which are the line segments that connect adjacent vertices.
We learn about diagonals of polygons in math classes because they are an important concept in geometry. Understanding diagonals can help us better understand the properties and characteristics of different types of polygons, such as triangles, quadrilaterals, and pentagons.
A fun fact about diagonals of polygons is that the number of diagonals in a polygon can be calculated using a simple formula. The number of diagonals in a polygon with n vertices is given by the equation: \(\displaystyle\frac{n(n-3)}{2}\).
Some related topics to diagonals of polygons include other concepts in geometry, such as angles, triangles, and quadrilaterals. Understanding diagonals can also help us better understand more advanced concepts in geometry, such as tessellations and non-Euclidean geometry.
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!