Practice Problems
State the domain of each rational function.
\(\textbf{1)}\) \(f(x)=\displaystyle\frac{x^3+2x^2}{x^2+3x+2} \)
\(\textbf{2)}\) \(f(x)=\displaystyle\frac{x^4+2x^3-15x^2}{x^2-3x} \)
\(\textbf{3)}\) \(f(x)=\displaystyle\frac{x^2-6x-40}{2x^2+3x-20} \)
\(\textbf{4)}\) \(f(x)=\displaystyle\frac{25-x^2}{x^2+4x-5} \)
\(\textbf{5)}\) \(f(x)=\displaystyle\frac{x^3+5x^2+3x+15}{x^3+3x} \)
\(\textbf{6)}\) \(f(x)=\displaystyle\frac{x^3-2x^2+x}{x^2-2x+1} \)
\(\textbf{7)}\) \(f(x)=\displaystyle\frac{x^2-1}{x-1} \)
\(\textbf{8)}\) \(f(x)=\displaystyle\frac{x^3-1}{x^2-1} \)
See Related Pages\(\)
\(\bullet\text{ Graphing Rational Functions}\)
\(\,\,\,\,\,\,\,\,f(x)= \displaystyle\frac{4x+5}{x+1}\,\,\)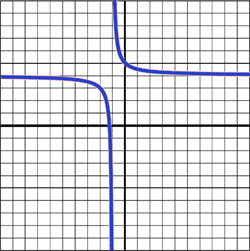
In Summary
The domain of a function is the set of all values of x for which the function is defined. In other words, it is the set of input values for which the function produces a valid output. A rational function is a function that can be expressed as the ratio of two polynomial functions. The domain of a rational function is determined by excluding the values of x that will create a division by zero error. On the graph these points show up as vertical asymptotes.
Rational functions are explored in depth in high school algebra or pre-calculus classes. There are many types of problems to do as you are learning about rational functions. You can do graphing, finding zeroes, solving for rational equations, solving for rational inequalities and more. These skills will help you in future classes to do much more.
Topics that use rational functions
Limits: Rational functions can be used to find the limit of a function as x approaches a particular value.
Differentiation: Rational functions can be differentiated using the quotient rule, which allows you to find the derivative of a function that is the ratio of two other functions.
Integration: Rational functions can be integrated using partial fractions, which involves decomposing the function into simpler fractions that can be integrated using basic integration techniques.
Optimization: Rational functions can be used to model real-world situations, such as finding the dimensions of a box with the maximum volume given a fixed surface area. These types of problems can be solved using calculus techniques such as optimization.
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!