Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?

Fibonacci Sequence as a Recursive Formula
\(a_1=0, a_2=1, a_n=a_{n-1}+a_{n-2}\)
See Related Pages\(\)
\(\bullet\text{ Fibonacci Sequence}\)
\(\,\,\,\,\,\,\,\,0,1,1,2,3,5,8,13,21…\)
\(\bullet\text{ Golden Ratio}\)
\(\,\,\,\,\,\,\,\,1.61803398875…\)
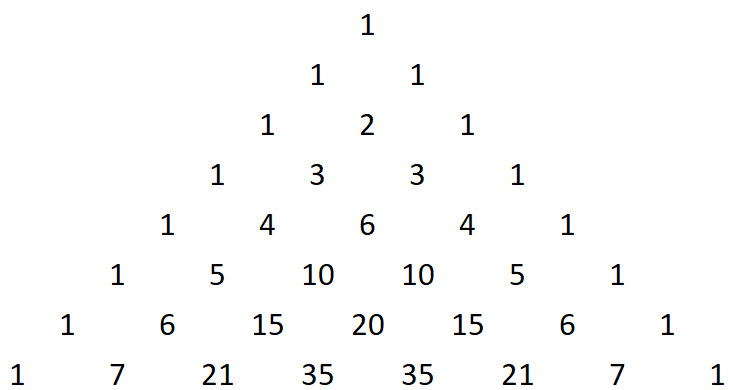
\(\bullet\text{ Pascal’s Triangle}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Binomial Theorem}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{n!}{(n-(k-1))!(k-1)!}a^{(n-(k-1)))b^{k-1}}\)
In Summary
The Fibonacci sequence is a series of numbers that follows a specific pattern. The first two numbers in the sequence are 0 and 1, and each subsequent number is the sum of the previous two. This pattern continues indefinitely, with the resulting sequence often referred to as the Fibonacci numbers. It can be represented by the formula F(n) = F(n-1) + F(n-2), where n is the position of the number in the sequence.
There are several reasons why we learn about the Fibonacci sequence. It appears in various areas of mathematics and science, such as algebra, geometry, and even biology. Understanding the Fibonacci sequence can help us solve problems and make predictions in these fields. Additionally, the Fibonacci sequence has a strong historical and cultural significance, as it was discovered by the famous mathematician Leonardo Fibonacci.
The Fibonacci sequence is typically introduced in a high school or college math class, such as algebra, precalculus, or calculus. However, it can also be introduced in a more advanced math class, such as discrete mathematics or number theory.
The ratio of consecutive numbers in the Fibonacci sequence approaches the golden ratio as the numbers get larger. The golden ratio, also known as the golden mean or the golden section, is a mathematical concept that represents a ratio of approximately 1.618:1. This ratio is often denoted by the Greek letter phi (φ). The golden ratio has been used by artists, architects, and designers throughout history because it is believed to be aesthetically pleasing.
The Fibonacci sequence can be found throughout nature. The arrangement of leaves on a stem, the branching of trees, and the spiral patterns in seashells and pinecones all exhibit the Fibonacci sequence. This is known as the Fibonacci spiral, and it is a result of the natural tendency for plants and animals to grow and reproduce in a way that is efficient..
The Fibonacci sequence was discovered by the Italian mathematician Leonardo Fibonacci, also known as Leonardo of Pisa. He introduced the sequence in his book “Liber Abaci,” which was published in the early 13th century. Fibonacci was inspired by the Hindu-Arabic numeral system and its use in commerce, and he used the Fibonacci sequence to solve problems related to finance and growth.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!