Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Notes
Problems & Videos
\(\textbf{1)}\) Write \(\log_{b}\frac{1}{5}=25\) in exponential form.

\(\textbf{2)}\) Write \(m^5=32\) in logarithmic form.

\(\textbf{3)}\) Write as a single logarithmic expression.
\(2\log_{5}(2)+\frac{1}{2}\log_{5}(x+3)-\log_{5}(x) \)

Expand the following logarithms.
\(\textbf{4)}\) \( \displaystyle\log_{b}(\frac{x^2z}{y^5}) \)

\(\textbf{5)}\) \( \displaystyle\log_{5}(\frac{\sqrt[3]{z}}{25y^5}) \)

\(\textbf{6)}\) \( \displaystyle\log_{2}(\frac{b\sqrt{n}}{32}) \)

Evaluate without using a calculator.
\(\textbf{7)}\) \( \log_{3}(1) \)

\(\textbf{8)}\) Use \(\log_{3}(2)\approx 0.6309\) and \(\log_{3}(7)\approx 1.7712\) to approximate \(\log_{3}(\frac{49}{2})\)

Approximate (calculator ok)
\(\textbf{9)}\) \( \log_{3}(5) \)

\(\textbf{10)}\) \( \log_{7}(34) \)
\(\textbf{11)}\) \( \log(2.3) \)

See Related Pages
\(\bullet\text{ Expanding Logarithms}\)
\(\,\,\,\,\,\,\,\,2\log_{b}(x)+\log_{b}(z)-5\log_{b}(y)…\)
\(\bullet\text{ Decibel Problems}\)
\(\,\,\,\,\,\,\,\,N_{dB}=10\log \left(\frac{P}{10^{-12}}\right)…\)
\(\bullet\text{ Earthquake Problems}\)
\(\,\,\,\,\,\,\,\,M=\log\frac{I}{10^{-4}}…\)
\(\bullet\text{ Domain and Range Logarithmic Functions}\)
\(\,\,\,\,\,\,\,\,f(x)=log(x) \rightarrow \text{Domain:} x\gt0… \)
\(\bullet\text{ Graphing Logarithmic Functions}\)
\(\,\,\,\,\,\,\,\,f(x)=log_{2}(x)\) 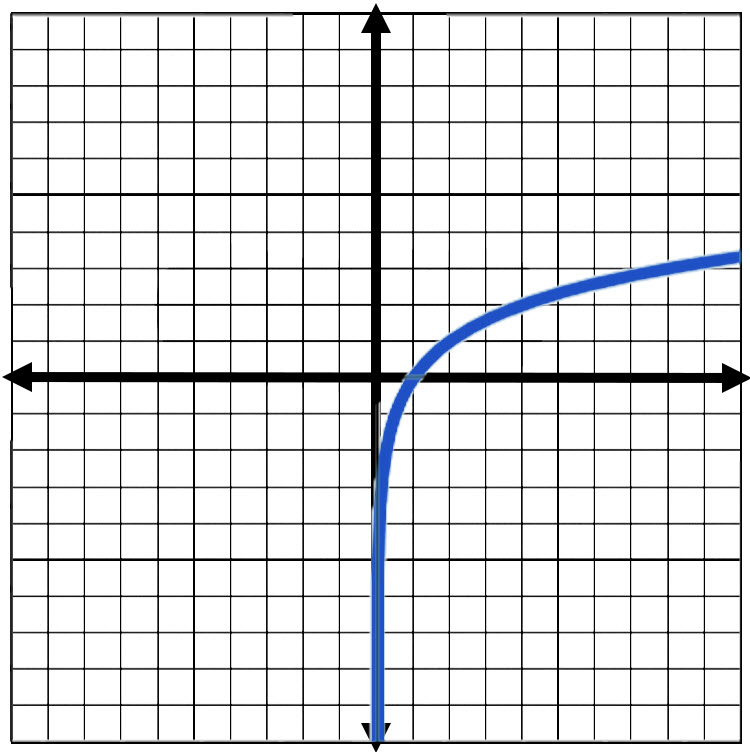
\(\bullet\text{ Solving Logarithmic Equations}\)
\(\,\,\,\,\,\,\,\,\log_{2}(5x)=\log_{2}(2x+12)…\)
\(\bullet\text{ Inverse of Logarithmic Functions}\)
\(\,\,\,\,\,\,\,\,f(x)=log_{2}(x) \rightarrow f^{-1}(x)=2^x\)
In Summary
Defining logarithms can be somewhat complex, but essentially they represent the exponent to which a base number must be raised in order to equal a given number. For example, the logarithm base 10 of 100 is 2, because 10 to the power of 2 equals 100.
There are several reasons why we learn about logarithms in algebra and precalculus classes. One of the main reasons is that they are often used in scientific and mathematical applications to solve problems involving exponential growth or decay. They are also used in finance, engineering, and other fields where it is necessary to understand how quantities change over time. Ultimately anytime the variable is in the exponent, we would us logarithms to solve for the variable.
Real world examples of Logarithms
Sound intensity: The intensity of a sound, or the loudness of a sound, is measured in decibels (dB). The decibel scale is logarithmic, which means that an increase of 10 dB represents a tenfold increase in sound intensity. For example, a sound that is 60 dB is ten times louder than a sound that is 50 dB.
Richter Scale or Earthquake magnitude: The magnitude of an earthquake is a measure of the energy released by the earthquake. The magnitude scale is logarithmic, which means that an increase of 1 magnitude unit represents a tenfold increase in energy. For example, an earthquake with a magnitude of 7 is ten times more powerful than an earthquake with a magnitude of 6.
pH of a solution: The pH of a solution is a measure of the acidity or basicity of the solution. The pH scale is logarithmic, which means that a change of 1 pH unit represents a tenfold change in acidity or basicity. For example, a solution with a pH of 4 is ten times more acidic than a solution with a pH of 5.
Stellar magnitude: The brightness of a star is measured in stellar magnitudes. The magnitude scale is logarithmic, which means that a difference of 1 magnitude unit represents a difference of about 2.5 times in brightness. For example, a star with a magnitude of 2 is about 2.5 times brighter than a star with a magnitude of 3.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!