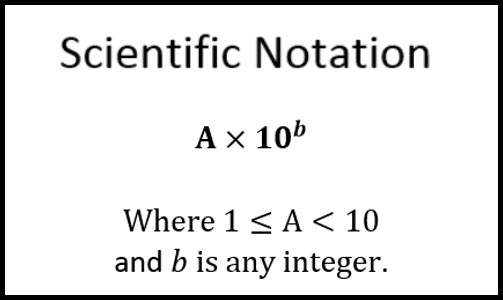
Scientific notation is a method for expressing numbers, and is the most convenient method for very large or very small numbers. Instead of writing the number in decimal form, like this,
\(3{,}285{,}000{,}000{,}000{,}000{,}000{,}000{,}000\)
you can write the number in Scientific Notation like this
\(3.285 \times 10^{24}\)
Same thing for very small numbers. Instead of this
\(0.000000000075\)
you can write the number in Scientific Notation like this
\(7.5 \times 10^{-11}\)
\(0.000000000075\)
you can write the number in Scientific Notation like this
\(7.5 \times 10^{-11}\)
Notes
Problems and Videos
Express in scientific notation
\(\textbf{1)}\) \( 125.78 \)

\(\textbf{2)}\) \( 0.00000456 \)

\(\textbf{3)}\) \( 1,892,000,000 \)

\(\textbf{4)}\) \( 13,405 \)

\(\textbf{5)}\) \( 0.00345 \)

Express in decimal form
\(\textbf{6)}\) \( 6.13×10^6 \)

\(\textbf{7)}\) \( 3.49×10^{-5} \)

\(\textbf{8)}\) \( 7.2×10^0 \)

Simplify, give answer in scientific notation
\(\textbf{9)}\) \( (5.8×10^4 )(3.8×10^3 ) \)

\(\textbf{10)}\) \( (5.8×10^{-4})(3.8×10^3) \)

\(\textbf{11)}\) \( (2.8×10^{-4})(1.8×10^{-3}) \)

\(\textbf{12)}\) \( \displaystyle\frac{1.6×10^5}{3.2×10^3} \)

\(\textbf{13)}\) \( (4.6×10^5)+(3.2×10^5) \)
\(\textbf{14)}\) \( (5.4×10^{-3})-(2.8×10^{-3}) \)
\(\textbf{15)}\) \( (1.6×10^5)+(3.2×10^3) \)
\(\textbf{16)}\) \( (1.6×10^5)-(3.2×10^4) \)
\(\textbf{17)}\) \( (5×10^5)+(3×10^4) \)
\(\textbf{18)}\) \( (6×10^7)-(4×10^5) \)
In Summary
Scientific notation is a way of expressing numbers that are too large or too small to be conveniently written in standard decimal form. In scientific notation, a number is written as the product of a number between 1 and 10, and a power of 10.
Scientific notation is typically introduced in middle or high school algebra classes, although it may also be covered in lower-level math classes depending on the curriculum. The concept of scientific notation is important for many higher-level math and science courses, including calculus and physics. It is also commonly used in many real-world applications, such as in engineering and scientific research.
It can be used to represent very large and very small numbers, even numbers that are too large or too small to be written in standard decimal form. For example, the number of atoms in the observable universe has been estimated to be around \(10^{80}\), which is a number too large to be written in standard decimal form. However, using scientific notation, this number can be written as \(1 \times 10^{80}\), which is much easier to understand and work with. Similarly, the size of the smallest particles known to science, such as quarks and neutrinos, is on the order of \(10^{-35}\) meters, which is also too small to be written in standard decimal form. However, using scientific notation, this number can be written as \(1 \times 10^{-35}\) meters, which is much easier to understand and work with.
There are many real-world examples of scientific notation being used in various fields. For instance, in engineering, scientific notation is often used to represent very large or very small measurements, such as the dimensions of a building or the weight of a vehicle. In scientific research, scientific notation is commonly used to represent large or small numbers, such as the size of a sample population or the concentration of a chemical solution. In finance, scientific notation is often used to represent very large or very small amounts of money, such as the value of a company or the interest rate on a loan. In astronomy, scientific notation is frequently used to represent very large or very small distances, such as the distance between galaxies or the size of a star. Overall, scientific notation is a useful and versatile tool for representing and working with very large or very small numbers in many different fields.
The concept of scientific notation is generally credited to the mathematician John Napier, who introduced the idea of using exponents to represent large numbers in his book “Mirifici Logarithmorum Canonis Descriptio” (Description of the Marvelous Canon of Logarithms) in 1614. However, the modern form of scientific notation, which uses a coefficient and a power of 10 to represent numbers, was developed later by other mathematicians, such as Simon Stevin in the 16th century and Leonhard Euler in the 18th century. The use of scientific notation became more widespread in the 19th and 20th centuries, as it was adopted by scientists and engineers for representing and working with very large or very small numbers in their research and calculations.
There are several topics that are related to scientific notation, including algebra, exponents, logarithms, and decimal notation.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!