Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Notes

Practice Problems
Find the derivative of each
\(\small{\textbf{1)}}\) Find \(f'(x)\) where \(f(x)=(3x+5)^4\)
\(\small{\textbf{2)}}\) Find \(f'(x)\) where \(f(x)=\sqrt{2x-1}\) 
\(\small{\textbf{3)}}\) Find \(f'(x)\) where \(f(x)=\displaystyle\frac{1}{x^3+3x-2}\) 
\(\small{\textbf{4)}}\) Find \(f'(x)\) where \(f(x)=x^2(2x-1)^3\) 
\(\small{\textbf{5)}}\) Find \(f'(x)\) where \(f(x)=\sqrt[3]{x^4-2x+1}\) 
\(\small{\textbf{6)}}\) Find \(f'(x)\) where \(f(x)=(x^3-2)^4\) 
\(\small{\textbf{7)}}\) Find \(f'(x)\) where \(f(x) = (3x^2 + 5)^6\)
\(\small{\textbf{8)}}\) Find \(f'(x)\) where \(f(x) = \displaystyle\frac{1}{\left( x^2 + 2x – 5 \right)^ 3}\)
\(\small{\textbf{9)}}\) Find \(f'(x)\) where \(f(x) = \sqrt[5]{{1 – x}}\)
\(\small{\textbf{10)}}\) Find \(f'(x)\) where \(f(x) = \csc \left( {2x} \right)\)
\(\small{\textbf{11)}}\) Find \(f'(x)\) where \(f(x) = 3\cos \left( {\tan \left( 4x \right)} \right)\)
\(\small{\textbf{12)}}\) Find \(f'(x)\) where \(f(x) = \tan \left( {15x + 14} \right)\)
\(\small{\textbf{13)}}\) Find \(f'(x)\) where \(f(x) = e^{(x^2 + 2)}\)
\(\small{\textbf{14)}}\) Find \(f'(x)\) where \(f(x) = e^{1 – \sin \left( x \right)}\)
\(\small{\textbf{15)}}\) Find \(f'(x)\) where \(f(x) = {3^{1 + 4x}}\)
\(\small{\textbf{16)}}\) Find \(f'(x)\) where \(f(x) = {\tan ^{ – 1}}\left( {5x + 2} \right)\)
\(\small{\textbf{17)}}\) Find \(f'(x)\) where \(f(x) = \ln \left({2x^3 +3x +6} \right)\)
\(\small{\textbf{18)}}\) Find \(f'(x)\) where \(f(x) = \ln \left( {\sin \left( x \right) + \cos \left( x \right)} \right)\)
\(\small{\textbf{19)}}\) Find \(f'(x)\) where \(f(x) = \ln \left( {\tan \left( x \right) + \cot \left( x \right)} \right)\)
\(\small{\textbf{20)}}\) Find \(f'(x)\) where \(f(x) = {\sin ^2}\left( x \right) + \sin \left( {{x^2}} \right)\)
\(\small{\textbf{21)}}\) Find \(f'(x)\) where \(f(x) = {e^{ – x}}+e^x\)
\(\small{\textbf{22)}}\) Find \(f'(x)\) where \(f(x) = 2x^3 + \left(4x^5 – 6x \right)^7\)
\(\small{\textbf{23)}}\) Find \(f'(x)\) where \(f(x) = \ln \left( {\sin \left( x \right)} \right) – \sin \left( \ln x \right) \)
\(\small{\textbf{24)}}\) Find \(f'(x)\) where \(f(x) = x^2\ln(x^2)\)
\(\small{\textbf{25)}}\) Find \(f'(x)\) where \(f(x) = \sin \left( {2x} \right)\cos \left( {2x} \right)\)
\(\small{\textbf{26)}}\) Find \(f'(x)\) where \( f(x) = \sin \left( {\cos \left( {2x} \right)} \right)\)
\(\small{\textbf{27)}}\) Find \(f'(x)\) where \(f(x) = \displaystyle\frac{\sin {2x} }{\cos{2x}}\)
\(\small{\textbf{28)}}\) Find \(f'(x)\) where \(f(x) = \sin \left( e^x \right)\)
\(\small{\textbf{29)}}\) Find \(f'(x)\) where \(f(x) = \sqrt {\tan \left( {2x} \right)}\)
\(\small{\textbf{30)}}\) Find \(f'(x)\) where \(f(x) = {\tan ^3}\left( {{x^3}} \right)\)
\(\small{\textbf{31)}}\) Find \(f'(x)\) where \(f(x)=\ln(\ln x)\)
\(\small{\textbf{32)}}\) Find \(f'(x)\) where \(f(x)=\displaystyle 3^{\sin^4(x^3)}\)
\(\small{\textbf{33)}}\) Find \(f'(x)\) where \(f(x)=\displaystyle \sec{\left(\sin(x)\right)}\)
\(\small{\textbf{34)}}\) Find \(f'(x)\) where \(f(x)=\displaystyle \ln{\sqrt{x}}\)
\(\small{\textbf{35)}}\) Find \(f'(3)\) where \(f(x)=g(h(x))\)
| \(1\) | \(2\) | \(3\) | \(4\) | |
| \(9\) | \(4\) | \(6\) | \(2\) | |
| \(8\) | \(3\) | \(5\) | \(2\) | |
| \(1\) | \(3\) | \(4\) | \(2\) | |
| \(4\) | \(-2\) | \(7\) | \(3\) |
\(\small{\textbf{36)}}\) Find \(f'(1)\) where \(f(x)=g(h(x))\)
| \(1\) | \(2\) | \(3\) | \(4\) | |
| \(9\) | \(4\) | \(6\) | \(2\) | |
| \(8\) | \(3\) | \(5\) | \(2\) | |
| \(1\) | \(3\) | \(4\) | \(2\) | |
| \(4\) | \(-2\) | \(7\) | \(3\) |
\(\small{\textbf{37)}}\) Find \(f'(2)\) where \(f(x)=g(h(x))\)
| \(1\) | \(2\) | \(3\) | \(4\) | |
| \(9\) | \(4\) | \(6\) | \(2\) | |
| \(8\) | \(3\) | \(5\) | \(2\) | |
| \(1\) | \(3\) | \(4\) | \(2\) | |
| \(4\) | \(-2\) | \(7\) | \(3\) |
\(\small{\textbf{38)}}\) Find \(f'(4)\) where \(f(x)=g(h(x))\)
| \(1\) | \(2\) | \(3\) | \(4\) | |
| \(9\) | \(4\) | \(6\) | \(2\) | |
| \(8\) | \(3\) | \(5\) | \(2\) | |
| \(1\) | \(3\) | \(4\) | \(2\) | |
| \(4\) | \(-2\) | \(7\) | \(3\) |
See Related Pages\(\)
\(\bullet\text{ Calculus Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Definition of Derivative}\)
\(\,\,\,\,\,\,\,\, \displaystyle \lim_{\Delta x\to 0} \frac{f(x+ \Delta x)-f(x)}{\Delta x} \)
\(\bullet\text{ Equation of the Tangent Line}\)
\(\,\,\,\,\,\,\,\,f(x)=x^3+3x^2−x \text{ at the point } (2,18)\)
\(\bullet\text{ Derivatives- Constant Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(c)=0\)
\(\bullet\text{ Derivatives- Power Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(x^n)=nx^{n-1}\)
\(\bullet\text{ Derivatives- Constant Multiple Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(cf(x))=cf'(x)\)
\(\bullet\text{ Derivatives- Sum and Difference Rules}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(x) \pm g(x)]=f'(x) \pm g'(x)\)
\(\bullet\text{ Derivatives- Sin and Cos}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}sin(x)=cos(x)\)
\(\bullet\text{ Derivatives- Product Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(x) \cdot g(x)]=f(x) \cdot g'(x)+f'(x) \cdot g(x)\)
\(\bullet\text{ Derivatives- Quotient Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}\left[\displaystyle\frac{f(x)}{g(x)}\right]=\displaystyle\frac{g(x) \cdot f'(x)-f(x) \cdot g'(x)}{[g(x)]^2}\)
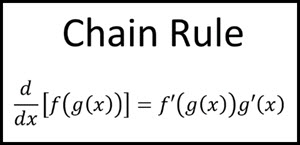
\(\bullet\text{ Derivatives- Chain Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(g(x))]= f'(g(x)) \cdot g'(x)\)
\(\bullet\text{ Derivatives- ln(x)}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[ln(x)]= \displaystyle \frac{1}{x}\)
\(\bullet\text{ Implicit Differentiation}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Horizontal Tangent Line}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Mean Value Theorem}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Related Rates}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Increasing and Decreasing Intervals}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Intervals of concave up and down}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Inflection Points}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Graph of f(x), f'(x) and f”(x)}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Newton’s Method}\)
\(\,\,\,\,\,\,\,\,x_{n+1}=x_n – \displaystyle \frac{f(x_n)}{f'(x_n)}\)
In Summary
The chain rule is a fundamental concept in calculus that allows us to differentiate composite functions.
The formal definition of the chain rule states that if we have a function \(f\left(g(x)\right)\), then the derivative of \(f\) with respect to \(x\) is equal to the derivative of \(f\) with respect to \(g\) multiplied by the derivative of \(g\) with respect to \(x\). This can be written as: \(f’\left(g(x)\right) = f'(g) \cdot g'(x)\).
Learning about the chain rule is important because it allows us to differentiate a wide variety of functions that would otherwise be difficult or impossible to differentiate. It is a key tool in the study of optimization and is widely used in fields such as physics, engineering, and economics.
The chain rule is taught in calculus courses. It is usually introduced after students have learned about the basic rules of differentiation, such as the power rule, product rule and quotient rule.
Real world examples of chain rule
The chain rule is often used in economics to analyze the effect of changes in one variable on another variable. For example, consider a firm that produces a good and sells it in a competitive market. The firm’s profit is a function of the price it can charge for the good, which is in turn a function of the market demand for the good. The chain rule can be used to analyze how a change in the market demand for the good affects the firm’s profit.
In biology, the chain rule is often used to analyze how changes in one variable affect another variable. For example, consider a population of animals that relies on a particular food source. The size of the population is a function of the availability of the food source, which is in turn a function of the environmental conditions. The chain rule can be used to analyze how a change in the environmental conditions affects the size of the population.
In engineering, the chain rule is often used to analyze the effect of changes in one variable on another variable. For example, consider a bridge that is designed to support a certain load. The load on the bridge is a function of the weight of the vehicles crossing the bridge, which is in turn a function of the number of vehicles crossing the bridge. The chain rule can be used to analyze how a change in the number of vehicles crossing the bridge affects the load on the bridge.
In meteorology, the chain rule is often used to analyze the effect of changes in one variable on another variable. For example, consider the temperature of the air in a particular location. The temperature of the air is a function of the amount of sunlight the location receives, which is in turn a function of the time of year. The chain rule can be used to analyze how a change in the time of year affects the temperature of the air in a particular location.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!