Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?

Lesson

Notes

Practice Problems

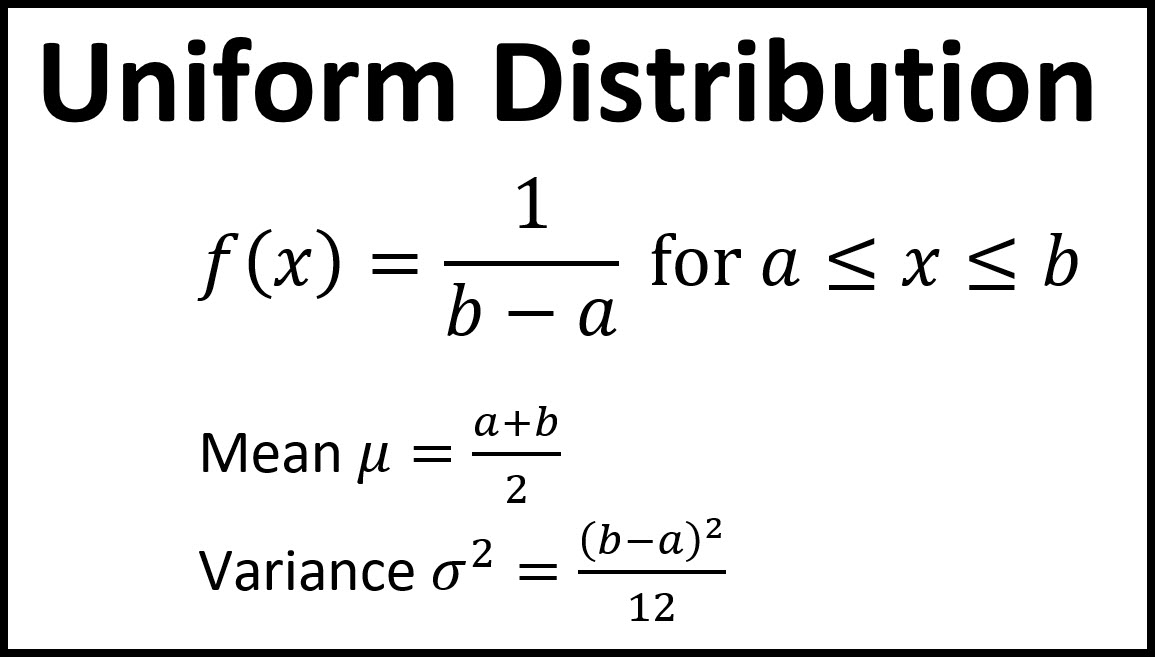
\(\textbf{1)}\) The trains stop at Andytown station every twenty minutes. Assume the distribution of the waiting time is a uniform distribution. Find the height of this uniform distribution.

Show Height

\(\text{height}=\displaystyle\frac{1}{20}\)

Show Work

\(\text{base} \times \text{height} = \text{Area}\)

\(\left(b-a\right) \times \text{height} = 1\)

\(\left(20-0\right) \times \text{height} = 1\)

\(\text{height}=\displaystyle\frac{1}{20}\)

\(\textbf{2)}\) The trains stop at Andytown station every twenty minutes. Assume the distribution of the waiting time is a uniform distribution. Find the probability of waiting more than 5 minutes. \(P(x \gt 5)\)

Show Answer

The answer is \(\displaystyle\frac{15}{20}=75\%\)

\(\textbf{3)}\) The trains stop at Andytown station every twenty minutes. Assume the distribution of the waiting time is a uniform distribution. Find the probability of waiting between 5 and 15 minutes. \(P(5\lt x \lt 15)\)

Show Answer

The answer is \(\displaystyle\frac{10}{20}=50\%\)

\(\textbf{4)}\) The trains stop at Andytown station every twenty minutes. Assume the distribution of the waiting time is a uniform distribution. Find the mean wait time.

Show Answer

The answer is 10 minutes

Show Work

\(\,\,\,\,\,\,\text{Mean}=\displaystyle \frac{a+b}{2}\)

\(\,\,\,\,\,\,\text{Mean}=\displaystyle \frac{0+20}{2}\)

\(\,\,\,\,\,\,\text{Mean}=\displaystyle \frac{20}{2}\)

\(\,\,\,\,\,\,10 \text{ minutes}\)

\(\textbf{5)}\) The trains stop at Andytown station every twenty minutes. Assume the distribution of the waiting time is a uniform distribution. Find the variance of the wait time.

Show Answer

The answer is \(33\frac{1}{3}\) minutes

Show Work

\(\,\,\,\,\,\,\text{Variance} = \sigma^2 = \displaystyle \frac {\left(b-a\right)^2}{12}\)

\(\,\,\,\,\,\,\sigma^2 = \displaystyle \frac {\left(20-0\right)^2}{12}\)

\(\,\,\,\,\,\,\sigma^2 = \displaystyle \frac {\left(20\right)^2}{12}\)

\(\,\,\,\,\,\,\sigma^2 = \displaystyle \frac {400}{12}\)

\(\,\,\,\,\,\,\)The answer is \(33\frac{1}{3}\) minutes

See Related Pages\(\)

\(\bullet\text{ Statistics Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)

\(\bullet\text{ Binomial Distribution}\)
\(\,\,\,\,\,\,\,\,p(r)={}_{n}C_{r}(p)^r(1-p)^{n-r}…\)

\(\bullet\text{ Poisson Distribution}\)
\(\,\,\,\,\,\,\,\,P(x)=\displaystyle\frac{\lambda^x e^{-\lambda}}{x!}…\)

\(\bullet\text{ Geometric Distribution}\)
\(\,\,\,\,\,\,\,\,P(X=n)=p(1-p)^{n-1}…\)

\(\bullet\text{ Continuity Correction}\)
\(\,\,\,\,\,\,\,\,c-.5\lt x\lt c+.5…\)

In Summary

The Uniform Distribution is a type of probability distribution where all outcomes are equally likely to occur. This means that the probability of each outcome is the same within a defined range or set of values.

Understanding uniform distributions is important in a variety of fields, including economics, engineering, and computer science. For example, uniform distributions are often used to model the outcomes of random events, such as rolling a die or drawing a card from a deck.

Uniform distributions are typically covered in probability and statistics courses.

The uniform distribution is sometimes referred to as the “rectangular distribution” because its probability density function (PDF) is shaped like a rectangle. This is in contrast to other probability distributions, such as the normal distribution, which have a bell-shaped PDF.

The concept of a uniform distribution has a long history, with roots dating back to ancient civilizations. However, the modern mathematical formulation of the uniform distribution is often attributed to 18th-century mathematician and astronomer Pierre-Simon Laplace.

Related topics to uniform distribution: Uniform distributions are closely related to other probability distributions, such as the binomial distribution and the normal distribution. Understanding these distributions can help you better understand how probability works in different contexts, and how to analyze and interpret data.

Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.

Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.

Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!

Thank you for visiting. How exciting!