Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Notes
Practice Problems
You are playing tennis with a friend. The probability you win each game is 40%.
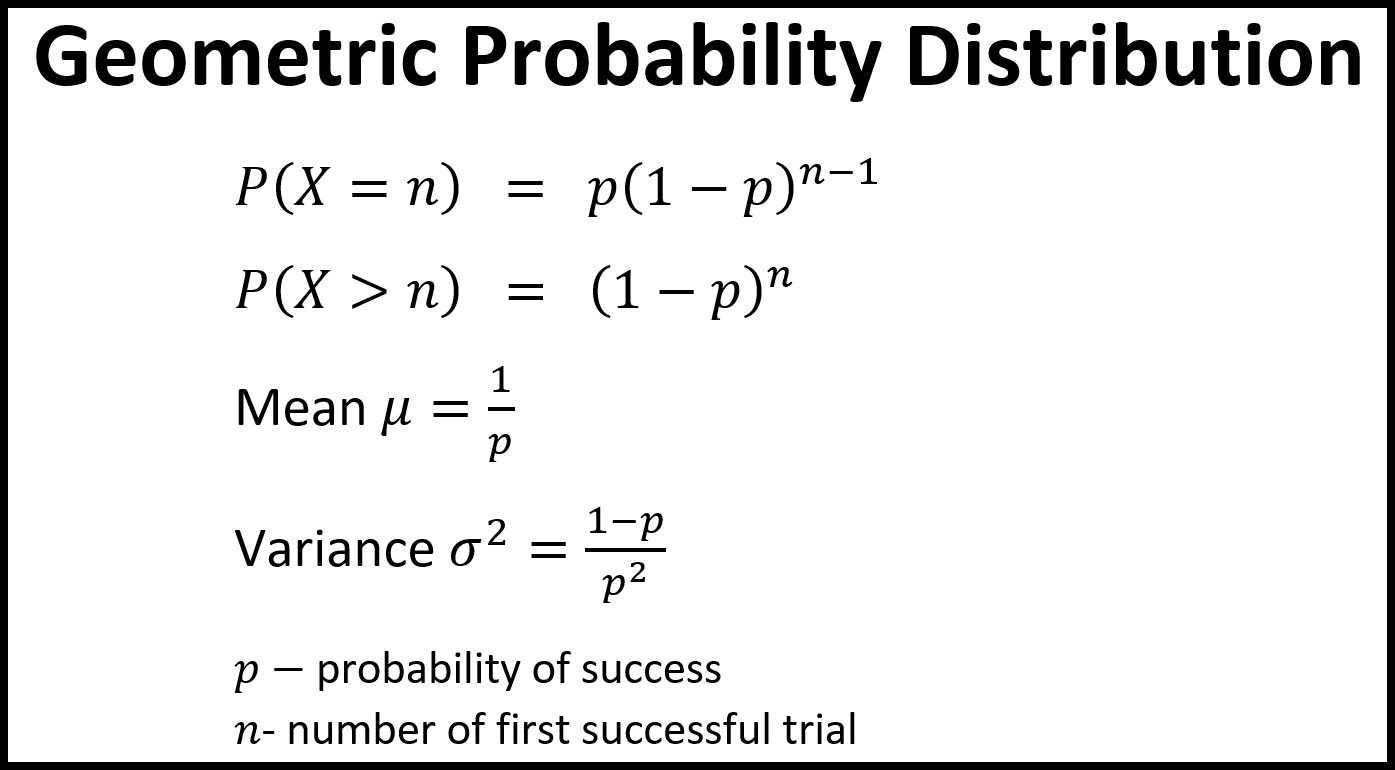
\(\textbf{1)}\) What is the probability your first win is on the 3rd game?
\(\textbf{2)}\) What is the probability your first win is on the 5th game?
\(\textbf{3)}\) What is the probability your first win is on the 1st game?
\(\textbf{4)}\) What is the mean number of games until your first win?
You are rolling a fair 6-sided die. The probability you roll a 5 is \(\frac{1}{6}\).
\(\textbf{5)}\) What is the probability your first “5” is on the 3rd roll?
\(\textbf{6)}\) What is the probability your first “5” is on the 5th roll?
\(\textbf{7)}\) What is the probability your first “5” is on the 1st roll?
\(\textbf{8)}\) What is the mean number of rolls until your first “5”?
See Related Pages\(\)
\(\bullet\text{ Statistics Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Uniform Distribution}\)
\(\,\,\,\,\,\,\,\,p(x)=\frac{1}{b-a}…\)
\(\bullet\text{ Binomial Distribution}\)
\(\,\,\,\,\,\,\,\,p(r)={}_{n}C_{r}(p)^r(1-p)^{n-r}…\)
\(\bullet\text{ Poisson Distribution}\)
\(\,\,\,\,\,\,\,\,P(x)=\displaystyle\frac{\lambda^x e^{-\lambda}}{x!}…\)
\(\bullet\text{ Continuity Correction}\)
\(\,\,\,\,\,\,\,\,c-.5\lt x\lt c+.5…\)
In Summary
The geometric distribution is a statistical model that describes the number of failures that occur before the first success in a series of independent and identical Bernoulli trials. It is used in fields such as engineering, computer science, and economics.
Real world examples of Geometric Distribution
The number of times a customer must call a company before their issue is resolved: Each time a customer calls, there is a fixed probability that their issue will be resolved on the first call. If it is not resolved, they must call again and try their luck again, creating a geometric distribution.
The number of times a machine must be used before it fails: Each time a machine is used, there is a fixed probability that it will fail on that use. If it does not fail, it must be used again and the process is repeated, creating a geometric distribution.
The number of times a gambler must play a game of chance before winning: Each time a gambler plays a game of chance, there is a fixed probability that they will win on that play. If they do not win, they must play again and the process is repeated, creating a geometric distribution.
The number of times a salesperson must make a cold call before making a sale: Each time a salesperson makes a cold call, there is a fixed probability that they will make a sale on that call. If they do not make a sale, they must call again and the process is repeated, creating a geometric distribution.
The number of times a scientist must conduct an experiment before obtaining a successful result: Each time a scientist conducts an experiment, there is a fixed probability that they will obtain a successful result on that trial. If they do not obtain a successful result, they must conduct the experiment again and the process is repeated, creating a geometric distribution.
Topics that use geometric distribution
Reliability engineering: In reliability engineering, the geometric distribution is often used to model the number of failures or defects in a system before the first success or acceptable unit is observed. For example, a manufacturer might use the geometric distribution to model the number of defective products that are produced before the first non-defective product is produced on a production line.
Queueing theory: In queueing theory, the geometric distribution is often used to model the number of customers that arrive at a service facility before the first customer is served. For example, a bank might use the geometric distribution to model the number of customers that arrive at a teller window before the first customer is served.
Epidemiology: In epidemiology, the geometric distribution is often used to model the number of secondary infections caused by a single infected individual in a population. For example, a public health agency might use the geometric distribution to model the number of individuals that are infected by a single infected individual in an outbreak of a contagious disease.
Marketing: In marketing, the geometric distribution is often used to model the number of contacts with a customer before a sale is made. For example, a company might use the geometric distribution to model the number of marketing emails that are sent to a customer before the customer makes a purchase.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!