Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?

Proofs

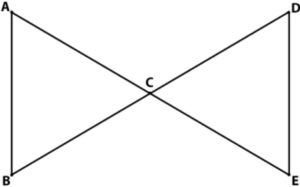
\(\textbf{1)}\) Given: \(C\) bisects \( \overline{AE} \) and \( \overline{BD} \)
\(\,\,\,\,\,\,\,\,\,\)Prove: \( \overline{AB} \cong \overline{DE}\)
\(\,\,\,\,\,\,\,\,\,\)

Show Proof Steps

\(\underline{\text{Statement}}\)	\(\underline{\text{Reason}}\)
\(C\) bisects \( \overline{AE} \) and \( \overline{BD} \)	Given
\( \overline{AC} \cong \overline{CE}; \overline{BC} \cong \overline{CD}\)	Definition of bisects
\(\angle ACB \cong \angle ECD\)	Vertical Angles
\(\triangle ACB \cong \triangle ECD\)	SAS triangle congruence
\( \overline{AB} \cong \overline{DE}\)	CPCTC

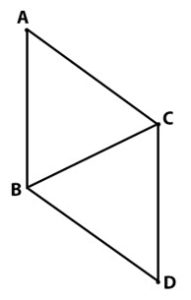
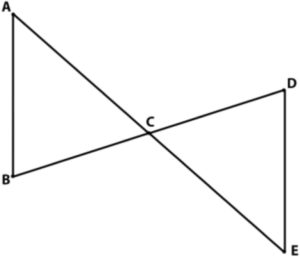
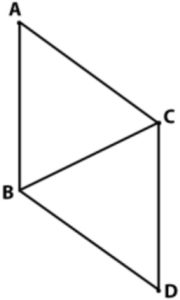
\(\textbf{2)}\) Given: \( \overline{AB} \parallel \overline{CD}\), \( \, \, \overline{AC} \parallel \overline{BD} \)
\(\,\,\,\,\,\,\,\,\,\)Prove: \( \angle A \cong \angle D\)
\(\,\,\,\,\,\,\,\,\,\)

Show Proof Steps

\(\underline{\text{Statement}}\)	\(\underline{\text{Reason}}\)
\( \overline{AB} \parallel \overline{CD}\)\( \overline{AC} \parallel \overline{BD} \)	Given
\(\angle ACB \cong \angle DBC\)\(\angle ABC \cong \angle DCB\)	Alternate Interior Angles
\( \overline{BC} \cong \overline{CB}\)	Reflexive
\(\triangle ABC \cong \triangle DCB\)	ASA triangle congruence
\(\angle A \cong \angle D\)	CPCTC

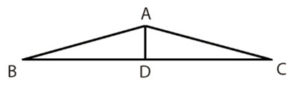
\(\textbf{3)}\) Given: \( \overline{AB} \cong \overline{AC}\), \( \, \, \overline{AD}\) bisects \( \overline{BC} \)
\(\,\,\,\,\,\,\,\,\,\)Prove: \(\angle B \cong \angle C\)
\(\,\,\,\,\,\,\,\,\,\)

Show Proof Steps

\(\underline{\text{Statement}}\)	\(\underline{\text{Reason}}\)
\( \overline{AB} \cong \overline{AC}\) \(\overline{AD}\) bisects \( \overline{BC} \)	Given
\( \overline{BD} \cong \overline{CD}\)	Definition of bisects
\( \overline{AD} \cong \overline{AD}\)	Reflexive
\(\triangle BDA \cong \triangle CDA\)	SSS triangle congruence
\(\angle B \cong \angle C\)	CPCTC

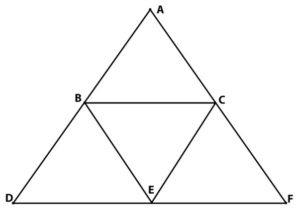
\(\textbf{4)}\) Given: \( \overline{AD} \cong \overline{AF}\), \( \, \, \overline{BD} \cong \overline{CF} \), \( \, \, E\) bisects \( \overline{DF} \)
\(\,\,\,\,\,\,\,\,\,\)Prove: \( \angle DEB \cong \angle FEC \)
\(\,\,\,\,\,\,\,\,\,\)

Show Proof Steps

\(\underline{\text{Statement}}\)	\(\underline{\text{Reason}}\)
\( \overline{AD} \cong \overline{AF}\) \(\overline{BD} \cong \overline{CF} \) \(E\) bisects \( \overline{DF} \)	Given
\(\angle D \cong \angle F\)	Isosceles Triangles Base Angles Theorem
\( \overline{DE} \cong \overline{FE}\)	Definition of bisects
\( \triangle DEB \cong \triangle FEC \)	SAS triangle congruence
\( \angle DEB \cong \angle FEC \)	CPCTC

See Related Pages\(\)

\(\bullet\text{ Geometry Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)

\(\bullet\text{ Proving Triangle Congruence SAS}\)
\(\,\,\,\,\,\,\,\,\)\(…\)

\(\bullet\text{ Proving Triangle Congruence SSS}\)
\(\,\,\,\,\,\,\,\,\) \(…\)

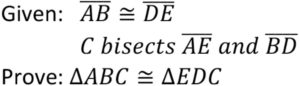
\(\bullet\text{ Proving Triangle Congruence ASA}\)
\(\,\,\,\,\,\,\,\,\) \(…\)

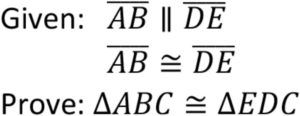
\(\bullet\text{ Proving Triangle Congruence AAS}\)
\(\,\,\,\,\,\,\,\,\) \(…\)

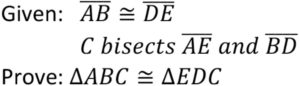
In Summary

Proving triangle congruence using CPCTC, or “corresponding parts of congruent triangles are congruent” theorem, is a key concept in geometry. Once we know that two triangles are congruent, we can conclude that any previously unknown corresponding parts must also be congruent.

Using CPCTC in proofs is typically covered in a high school geometry class.

CPCTC will only work if 2 triangles are know to be congruent. Therefore proofs using CPCTC usually also contain at least one of the following theorems, SSS, SAS, ASA, AAS, or HL. These theorems prove the congruence of triangles and are often used in the step prior to CPCTC.

About Andymath.com

Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.

Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.

Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!

Thank you for visiting. How exciting!