Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Notes
Practice Problems
\(\textbf{1)}\) Graph \( \displaystyle \frac{x^2}{4}+\frac{y^2}{25}=1 \)
\(\textbf{2)}\) Graph \( \displaystyle \frac{(x-3)^2}{16}-\frac{(y+2)^2}{4}=1 \)
\(\textbf{3)}\) Find the center of the hyperbola \(\displaystyle\frac{(x-3)^2}{16}-\frac{(y+2)^2}{4}=1\).
\(\textbf{4)}\) Find the vertices of the hyperbola \(\displaystyle\frac{(x-3)^2}{16}-\frac{(y+2)^2}{4}=1\).
\(\textbf{5)}\) Find the Foci of the hyperbola \(\displaystyle\frac{(x-3)^2}{16}-\frac{(y+2)^2}{4}=1\).
\(\textbf{6)}\) Find the slopes of the asymptotes of the hyperbola \(\displaystyle\frac{(x-3)^2}{16}-\frac{(y+2)^2}{4}=1\).
\(\textbf{7)}\) Find the eccentricity of the hyperbola \(\displaystyle\frac{(x-3)^2}{16}-\frac{(y+2)^2}{4}=1\).
\(\textbf{8)}\) Find the equation of the hyperbola with vertices \((3,4)\) and \((9,4)\) and Foci \((1,4)\) and \((11,4)\).
\(\textbf{9)}\) Use completing the square to rewrite the equation in standard form. \( x^2+4x=25y^2+250y+646 \)
See Related Pages\(\)
\(\bullet\text{ Hyperbola Calculator }\)
\(\,\,\,\,\,\,\,\,\text{(emathhelp.net)}\)
\(\bullet\text{ Hyperbola Grapher }\)
\(\,\,\,\,\,\,\,\,\text{(Desmos.com)}\)
\(\bullet\text{ All Conic Section Notes}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Equation of a Circle}\)
\(\,\,\,\,\,\,\,\,(x-h)^2+(y-k)^2=r^2…\)
\(\bullet\text{ Parabolas}\)
\(\,\,\,\,\,\,\,\,y=a(x-h)^2+k…\)
\(\bullet\text{ Axis of Symmetry}\)
\(\,\,\,\,\,\,\,\,x=-\frac{b}{2a}…\)
\(\bullet\text{ Ellipses}\)
\(\,\,\,\,\,\,\,\,\frac{(x-h)^2}{a^2}+\frac{(y-k)^2}{b^2}=1…\)
\(\bullet\text{ Area of Ellipses}\)
\(\,\,\,\,\,\,\,\,\text{Area}=\pi a b…\)
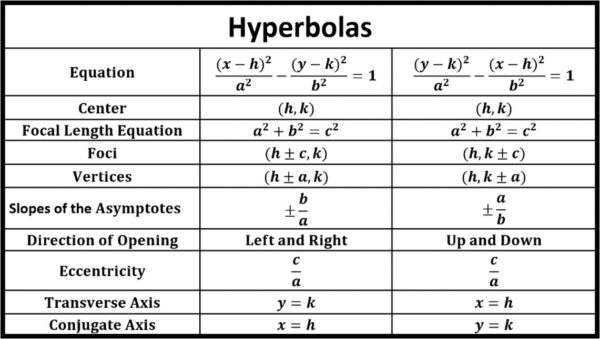
\(\bullet\text{ Hyperbolas}\)
\(\,\,\,\,\,\,\,\,\frac{(x-h)^2}{a^2}-\frac{(y-k)^2}{b^2}=1…\)
\(\bullet\text{ Conic Sections- Completing the Square}\)
\(\,\,\,\,\,\,\,\,x^2+8x+y^2−6y=11 \Rightarrow (x+4)^2+(y−3)^2=36…\)
\(\bullet\text{ Conic Sections- Parametric Equations}\)
\(\,\,\,\,\,\,\,\,x=h+r \cos{t}\)
\(\,\,\,\,\,\,\,\,y=k+r \sin{t}…\)
\(\bullet\text{ Degenerate Conics}\)
\(\,\,\,\,\,\,\,\,x^2−y^2=0…\)
\(\bullet\text{ Andymath Homepage}\)
In Summary
Hyperbolas are used in many different fields, including engineering, physics, and mathematics, to model and describe real-world phenomena. They are a type of geometric shape that consists of two branches, each of which is a curve that is shaped like a U or an inverted U. The branches of a hyperbola are mirror images of each other. When graphing a hyperbola it is important to identify these key parts, the vertices, the foci and the asymptotes.
Hyperbolas are conic sections. Conic secteions are curves created by the intersection of a plane and a cone. There are four types of conic sections: circles, ellipses, parabolas, and hyperbolas.
\(\cdot\)If the plane is perpendicular to the axis of the cone, the intersection of the plane and the cone will be a circle.
\(\cdot\)If the plane is between parallel and perpendicular to the axis of the cone, the intersection of the plane and the cone will be an ellipse.
\(\cdot\)If the plane is parallel to the axis of the cone, the intersection of the plane and the cone will be a parabola.
\(\cdot\)If the plane is beyond parallel to the axis of the cone, the intersection of the plane and the cone will be a hyperbola.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!
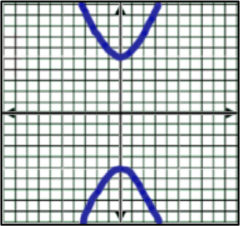 see video for details
see video for details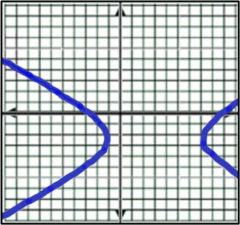 see video for details
see video for details