Parallel lines never intersect and therefore have the same slopes.
Perpendicular lines intersect at right angles and have negative reciprocal slopes.
There are notes, practice problems with solutions, videos, and related pages below!
Notes

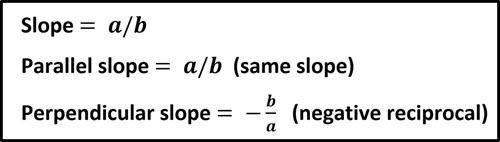
Problems & Videos
\(\textbf{1)}\) What is the equation of the line through \((2,4)\) and parallel to \(y=3x+5\)?

\(\textbf{2)}\) What is the equation of the line through \((2,4)\) and perpendicular to \(y=3x+5\)?

\(\textbf{3)}\) What is the equation of the line through \((5,6)\) and perpendicular to \(2x+6y=24\)?

\(\textbf{4)}\) Equation of line Perpendicular to \(x=3\) and contains point \((4,5)\)

\(\textbf{5)}\) What is the equation of the line through \((8,-3)\) and perpendicular to \(y=4x+3\)?
\(\textbf{6)}\) What is the equation of the line through \((7,30)\) and parallel to \(y=5x-2\)?
Are the following pairs of lines parallel, perpendicular or neither?
\(\textbf{7)}\) \(y=3x+2\)
\(\hspace{11pt}y=3x-4\)
\(\textbf{8)}\) \(y=\frac{1}{3}x+12\)
\(\hspace{11pt}y=-\frac{1}{3}x-2\)
\(\textbf{9)}\) \(y=\frac{2}{3}x+4\)
\(\hspace{11pt}y=-\frac{3}{2}x+1\)
\(\textbf{10)}\) \(y=-5x+3\)
\(\hspace{11pt}y=\frac{1}{5}x+6\)
\(\textbf{11)}\) \(x+4y=12\)
\(\hspace{11pt}x+4y=-2\)
\(\textbf{12)}\) \(y=3\)
\(\hspace{16pt}x=4\)
\(\textbf{13)}\) \(y=4\)
\(\hspace{16pt}y=-\frac{1}{4}\)
\(\textbf{14)}\) \(x+4y=4\)
\(\hspace{16pt}4x-y=6\)
Challenge Problems
\(\textbf{15)}\) Find the x intercept of the line through \((0,4)\) and parallel to \(y-2x=4\)?
See Related Pages\(\)
\(\bullet\text{ Geometry Homepage}\)
\(\,\,\,\,\,\,\,\,\text{All the Best Topics…}\)
\(\bullet\text{ Graphing Linear Equations}\)
\(\,\,\,\,\,\,\,\,2x-3y=6 \) 
\(\bullet\text{ Slope Formula}\)
\(\,\,\,\,\,\,\,\,m=\frac{y_2-y_1}{x_2-x_1}\)
\(\bullet\text{ Net Change}\)
\(\,\,\,\,\,\,\,\,y_2-y_1\)
\(\bullet\text{ Slope Intercept Form}\)
\(\,\,\,\,\,\,\,\,y=mx+b\)
\(\bullet\text{ Point Slope Form}\)
\(\,\,\,\,\,\,\,\,y-y_1=m(x-x_1)\)
\(\bullet\text{ Distance Between a Point and a Line}\)
\(\,\,\,\,\,\,\,\,(3,4) \text{ and } y=\frac{3}{4}x−2\)
\(\bullet\text{ Finding x- and y- intercepts}\)
\(\,\,\,\,\,\,\,\,y=2x+4\)
In Summary
The slope of a line is a measure of its steepness, typically thought of as rise/run. The slope of a line is positive if the line slopes upward from left to right, and it is negative if the line slopes downward from left to right.
Parallel lines have the same slope, and perpendicular lines have slopes that are negative reciprocals of each other.
Parallel and perpendicular slopes are typically covered in a high school geometry or algebra class.
Parallel and perpendicular slopes have many real-world applications. For example, they can be used to analyze the structural stability of buildings and bridges, or to calculate distances and angles in surveying and navigation.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!