Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?
Questions & Solutions
\(\textbf{1)}\) What is the complex conjugate of \(3i+4\)
\(\textbf{2)}\) What is the complex conjugate of \(i-5\)
\(\textbf{3)}\) What is the complex conjugate of \(6i-2\)
Use the complex conjugate of the denominator to simplify
\(\textbf{4)}\) Simplify \( \displaystyle\frac{2i}{3+4i} \)
\(\textbf{5)}\) Simplify \( \displaystyle\frac{6+i}{3+2i} \)
\(\textbf{6)}\) Simplify \( \displaystyle\frac{3-i}{5+3i} \)
\(\textbf{7)}\) Simplify \( \displaystyle\frac{3-2i}{3-i} \)
\(\textbf{8)}\) Simplify \( \displaystyle\frac{i}{9-2i} \)
See Related Pages\(\)
\(\bullet\text{ Complex Numbers Calculator (Symbolab.com)}\)
\(\)
\(\bullet\text{ Adding and Subtracting Polynomials}\)
\(\,\,\,\,\,\,\,\,(4d+7)−(2d−5)…\)
\(\bullet\text{ Multiplying Polynomials}\)
\(\,\,\,\,\,\,\,\,(x+2)(x^2+3x−5)…\)
\(\bullet\text{ Dividing Polynomials}\)
\(\,\,\,\,\,\,\,\,(x^3-8)÷(x-2)…\)
\(\bullet\text{ Dividing Polynomials (Synthetic Division)}\)
\(\,\,\,\,\,\,\,\,(x^3-8)÷(x-2)…\)
\(\bullet\text{ Synthetic Substitution}\)
\(\,\,\,\,\,\,\,\,f(x)=4x^4−3x^2+8x−2…\)
\(\bullet\text{ End Behavior}\)
\(\,\,\,\,\,\,\,\, \text{As } x\rightarrow \infty, \quad f(x)\rightarrow \infty \)
\(\,\,\,\,\,\,\,\, \text{As } x\rightarrow -\infty, \quad f(x)\rightarrow \infty… \)
\(\bullet\text{ Completing the Square}\)
\(\,\,\,\,\,\,\,\,x^2+10x−24=0…\)
\(\bullet\text{ Quadratic Formula and the Discriminant}\)
\(\,\,\,\,\,\,\,\,x=-b \pm \displaystyle\frac{\sqrt{b^2-4ac}}{2a}…\)
\(\bullet\text{ Multiplicity of Roots}\)
\(\,\,\,\,\,\,\,\,\)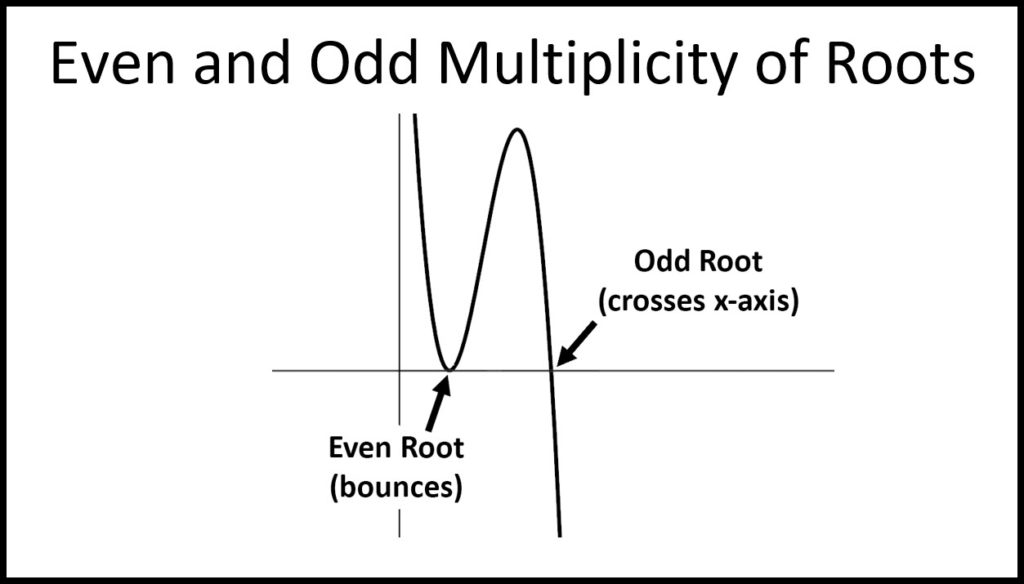 \(…\)
\(…\)
\(\bullet\text{ Rational Zero Theorem}\)
\(\,\,\,\,\,\,\,\, \pm 1,\pm 2,\pm 3,\pm 4,\pm 6,\pm 12…\)
\(\bullet\text{ Descartes Rule of Signs}\)
\(\,\)
\(\bullet\text{ Roots and Zeroes}\)
\(\,\,\,\,\,\,\,\,\text{Solve for }x. 3x^2+4x=0…\)
\(\bullet\text{ Linear Factored Form}\)
\(\,\,\,\,\,\,\,\,f(x)=(x+4)(x+1)(x−3)…\)
\(\bullet\text{ Polynomial Inequalities}\)
\(\,\,\,\,\,\,\,\,x^3-4x^2-4x+16 \gt 0…\)
\(\bullet\text{ Andymath Homepage}\)
In Summary
The complex conjugate of a complex number is a number that is obtained by changing the sign of the imaginary part of the number. For example, the complex conjugate of 4 + 3i is 4 – 3i, and the complex conjugate of 2 – 5i is 2 + 5i.
Complex conjugates are important in complex analysis, because they have many useful properties. For example, the product of a complex number and its complex conjugate is always a real number. If you multiply a complex number by its complex conjugate, the imaginary part of the result will be 0, so the result will be a real number.
Complex conjugates can also be used to simplify the division of complex numbers. When you divide complex numbers, you can multiply the numerator and denominator by the conjugate of the denominator, which has the effect of “canceling out” the imaginary part of the denominator. This makes the division much easier to perform, because it reduces the number of imaginary parts in the expression.
Complex conjugates are typically introduced in an algebra 2 course on complex numbers. Students learn about the properties of complex numbers, including how to add, subtract, multiply, and divide them, as well as how to find their conjugates. In general, complex conjugates are an important concept in complex analysis, and they have many useful properties that make working with complex numbers easier and more convenient. Complex conjugates have many real-world applications, particularly in the fields of engineering, physics, and electrical engineering.
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at tutoring@andymath.com. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at tutoring@andymath.com for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!